A rapid method for estimating the median diameter of the stem profile of Norway spruce (Picea abies Karst) trees
iForest - Biogeosciences and Forestry, Volume 10, Issue 1, Pages 328-333 (2017)
doi: https://doi.org/10.3832/ifor1945-009
Published: Feb 11, 2017 - Copyright © 2017 SISEF
Research Articles
Abstract
The median diameter of a longitudinal section of the stem may be used to determine the stem volume. However, to calculate stem volume, many measurements of diameter at different heights along the stem are required. Therefore, this approach is not generally applied because time-consuming and expensive. Here, we propose a novel, more rapid method to obtain median diameter using the area of the stem profile. A total of 218 height/diameter classes from more than 5000 spruce trees (Picea abies Karst.) were used to compute the median diameter using the classical method. In parallel, a regression model to assess the median diameter was developed. The strongest predictor of the median diameter for the stem profile was the diameter at breast height (R2 = 0.9985). Statistical analysis revealed that the height of the median diameter on the stem profile was 0.3 × H (tree height). The model was verified on standing and felled trees, revealing that differences between classical computations and the proposed model were less than 2% in most cases (86.24% of trees). The median diameter of the stem profile provides valuable information on stand architecture that could help in advancing our understanding on the mechanical stability of Norway spruce trees (i.e., delineating breakage point), growth model predictions, and competition among trees.
Keywords
Forest Mensuration, Median Diameter, Stem, Structure, Tree Stability
Introduction
Various methods are used to estimate the volume of both standing and felled trees. Of the many methods applied to estimate the bole volume of felled trees, Smalian’s, Huber’s and Newton’s formulae are usually adopted. All three methods provide good results for a frustum of a paraboloid and a cylinder ([13], [15], [18], [19], [25], [14], [33], [36], [38], [29], [1]). For standing trees, different formulae with three parameters (breast height diameter, height, and form) are used based on several recommendations ([28], [26], [13], [3], [25], [16], [22], [14], [19], [33], [36]). Alternatively, stem diameter at the center of wind resistance may be used to compute volume. This diameter is located at the center of gravity of the tree crown. The relative height of this point above the ground was used by Jonson in 1928; however, this model was abandoned in favor of more efficient ways to estimate stem volume ([35]).
Nonetheless, some equations use sections other than the cross-sectional area at the midpoint, or the cross-sectional area at the lower and upper end of the bole ([10], [39], [42], [6], [40], [7], [21]). Hossfeld outlines the importance of the cross-sectional area at one-third of the stem length/height ([13], [18], [19], [36], [38], [8], [9]). A similar theory uses the true form quotient defined by diameters measured above the ground at 0.1 of tree height (H) and 0.3 × H ([14]).
If the median diameter value of the stem profile is known, the stem volume may be computed using the Mathiesen’s formula ([20] - eqn. 1):
where DM is the median diameter of the stem profile; and A is the total area of the stem profile (∑ Ai). The area of each section, Ai (L × Di) is based on L, the length of the stem sections, while Di represents the diameter at the middle of the sections i (i =1, 2, …, n). This method was proposed in 1925, with its principle considering the center of gravity of the median diameter in relation to the area of the stem profile. The median diameter is defined as the diameter that divides the stem profile into two equal parts of the area. However, because laborious calculation is required, Mathiesen’s formula is avoided in practice, with Huber’s formula being preferred to determine the precise volume of the tree stem ([17], [19]). The size of median diameter may be found from the area when the profile shape is known, using graphical softwares such as those used in geodesy ([31], [32]). However, in practice, it is difficult to measure the shape of the profile, making this method inefficient.
This study aimed to develop a more rapid method for assessing the median diameter of the stem profile of Norway spruce trees (Picea abies Karst.). Such a method allows the Mathiesen’s formula to become a feasible tool for practical use. In addition, because tree resistance is related to stem mass ([23], [34]), the median diameter computed for the area of the stem profile could be used to study tree resistance against the wind. Given the importance and forested area covered by Norway spruce in Romania (22% - [30]), the study focused on this tree species.
Materials and methods
Materials
A database was established in 2012 by compiling the stem diameters of 5403 Norway spruce trees from Romania, grouped into 218 diameter-height classes (Tab. S1 in Supplementary material). Original measurements were performed by the FRMPI (Forest Research and Management Planning Institute of Romania) before 1950 in 200 plots, located in the Romanian Carpathians. The database consists of Norway spruce trees with breast height diameter (DBH) between 12 and 60 cm, and total height between 10 and 42 m. These data were published in 1957 as “General tables on decrease of stem diameter” ([27]). For all 218 classes of trees, Vasilescu ([37]) computed (Tab. S1 in Supplementary material) the median diameter of the stem profile (Fig. 1) using sections of 2 m for the longitudinal section of the stem area (eqn. 2), the absolute height from the lower end of the trunk where median diameter is measured (HDM - eqn. 3), and the relative height from the lower end of the trunk where this diameter occurs (HDMrel - eqn. 4).
Fig. 1 - Position of the median diameter (DM) on the stem profile. (HDM): height of the median diameter along the stem; (A): area of the stem profile; (Ai): area of the i-th longitudinal section. DM divides the stem profile into two parts of equal area (A/2).
The median diameter of the stem profile (DM) may be obtained as (eqn. 2):
where ADM1 and ADM2 are the areas with values under and above A/2 in the row of the cumulated areas of the sections; DM1 and DM2 are the corresponding values for diameters of ADM1 and ADM2, respectively. The parameter HDM can be estimated using eqn. 3:
where HDM1 and HDM2 are the distances between the lower end of the trunk and the point where DM1 and DM2 occur. Consequently, HDMrel may be derived as follows (eqn. 4):
Data analysis
From the previous work ([37]), data for breast height diameters were grouped into 25 diameter classes of size 2 cm. Total heights were also assigned to classes of 2 m in size.
A correlation analysis was applied to test the association between the median diameter at breast height (DBH) and DM, HDM, and HDMrel. Similarly, the association of tree height (H) with DM, HDM, and HDMrel was also tested. Moreover, DBH and H were used as independent variables in regression analysis to establish a rapid method for estimating the median diameter. The best fitted models to express the relationship between median diameter or its height and tree size data were examined using the F (Fisher) test. The accuracy of the models for the median diameter of the stem profile was estimated by computing the bias as (eqn. 5):
The coefficient of variation within each breast height diameter class was computed for height, median diameter of the stem profile, and absolute and relative height of the median diameter, in order to assess data homogeneity.
Results and discussion
Relationship between median diameter and tree size
Fig. 2 - Regression analysis of the median diameter of the stem profile in Picea abies Karst. With: (a) breast height diameter; and (b) height. Best fitting models are shown by solid lines (F test: n = 218, P < 0.001).
The evolution of the median diameter of the stem profile as a function of breast height diameter for Norway spruce trees in Romania is shown in Fig. 2a. A significant, positive relationship was found by regression analysis (R2 = 0.9985; F = 143871.1; df = 1, 216; P < 0.0001), and the following regression model was obtained (eqn. 6):
The same values of median diameter grouped by total tree height indicated a large variation for each tree height class (Fig. 2b). Using pairs, defined by median diameter and tree height, the coefficient of determination (R2) was only 0.5523. The best fitted model in this case was a polynomial expression (eqn. 7):
(R2 = 0.5523; F = 132.6324; df = 2, 215; P < 0.0001). However, the model accuracy was lower than that obtained using eqn. 6.
Analysis of the absolute height of median diameter
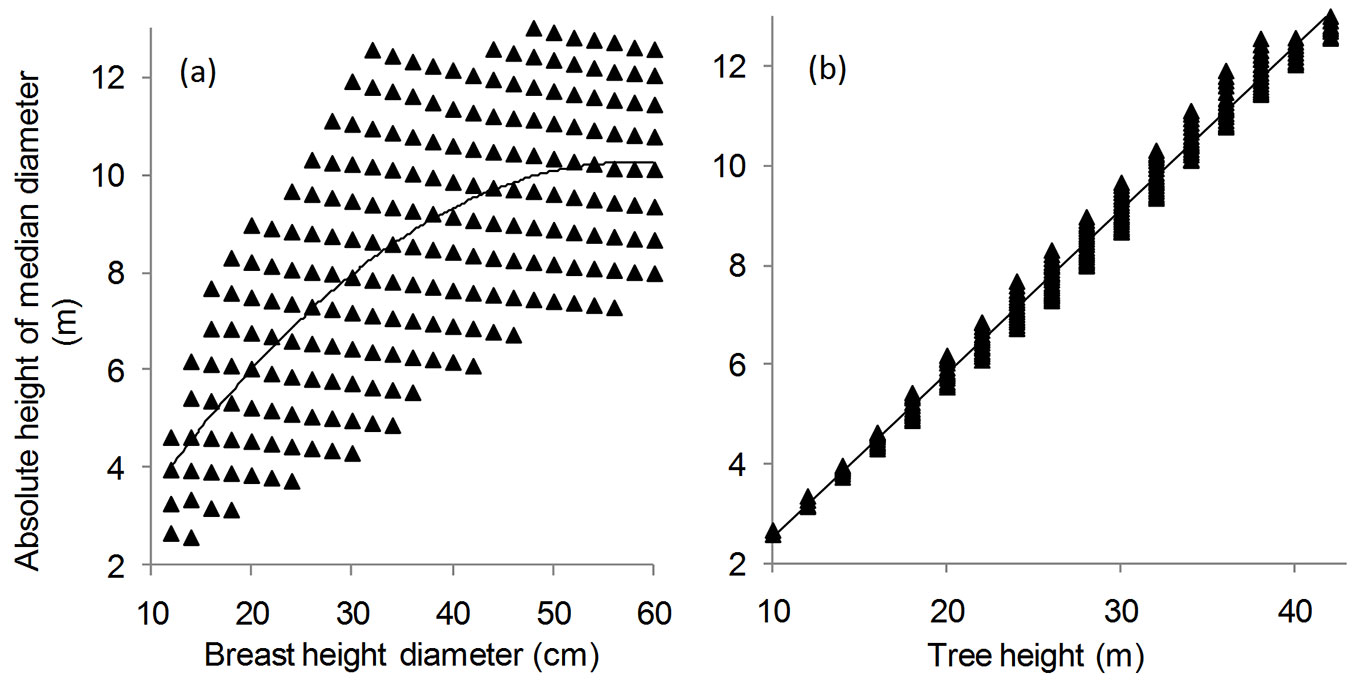
Fig. 3 - Relationship of the absolute height of the median diameter along the stem profile with: (a) breast height diameter; and (b) tree height. Best fitting models are shown by solid lines (F test: n = 218; P < 0.001).
The relationships of the absolute height of the median diameter with the diameter at breast height (DBH) and the total height (H) are shown in Fig. 3a and Fig. 3b, respectively. The polynomial regression applied indicated a weaker relationship in the first case (R2 = 0.4573; F = 90.5741; df = 2, 215; P < 0.0001) as compared with the second case (R2 = 0.9876; F = 17234.41; df = 1, 216; P < 0.0001). The best fitted models using DBH and H as predictors were as follows (eqn. 8 and eqn. 9, respectively):
The low accuracy of the first model (eqn. 8) was confirmed by the large dispersion of values of HDM, as depicted in Fig. 3a.
A positive correlation was detected between the absolute height of the median diameter and tree height (r = 0.9937).
Analysis of the relative height of median diameter
Fig. 4 - Values of the relative height of median diameter along the stem with respect to: (a) the class of breast height diameter; and (b) the tree height class. The best fitting model is shown by a solid line (F test: n = 218; P < 0.001), while the dashed line represents the horizontal asymptote, HDMrel = 0.2961 (F test: n = 218; P < 0.001).
The core of this study is the analysis of the relationship of the relative height of median diameter (HDMrel) with DBH and H in Norway spruce trees. Our results showed that the relative height along the stem profile where the median diameter is recorded was poorly correlated with the breast height diameter (Fig. 4a). HDMrel values were mainly distributed along and around a horizontal asymptote (intercept = 0.2961, t[216] = 103.0696, P < 0.0001), according to the following relationship (eqn. 10):
The mean HDMrel value computed using all the 218 tree size classes was 0.298695 ± 0.001936 (95% confidence interval), with a low coefficient of variation (4.88%), indicating fairly homogenous samples.
The results revealed that the median diameter of the longitudinal sections of the stem could be determined as approximately 0.3 × H in the case of Norway spruce trees in Romania.
The relationship between the relative height of the median diameter along the stem and total tree height (Fig. 4b) was modeled using a polynomial regression, obtaining the following best-fitting model (R2 = 0.5344; F = 123.4187; df = 2, 215; P < 0.0001 - eqn. 11):
Data variability
The coefficient of variation was computed for each breast height diameter class of each studied variable of the stem profile: tree height, median diameter of the longitudinal section, and absolute and relative height of median diameter.
The classes of diameter at breast height were homogenous for all the studied variables. The coefficient of variation was lower than 30% in most cases, ranging between 13.99% and 25.78% for total height, and between 15.73% and 31.64% for the absolute height of the median diameter on the stem. For comparison, the median diameter values (range: 0.60% to 1.64%) and the relative location of median diameter values (range: 2.04% to 6.74%) were significantly lower. Due to high data homogeneity, arithmetic means of the DBH classes were used. In this case, the arithmetic mean of all HDMrel mean values was 0.2978. The observed variation of values in all 25 breast height diameter classes was extremely low (coefficient of variation = 1.99%).
Accuracy of predictive models
The median diameter of the stem profile assessed using DBH (eqn. 6) was compared with the “true” median diameter using classical methods (eqn. 2), obtaining a maximum absolute difference of 0.88 cm. For 57.34% of the analyzed trees, even a lower difference was observed (between -3 and 3 mm).
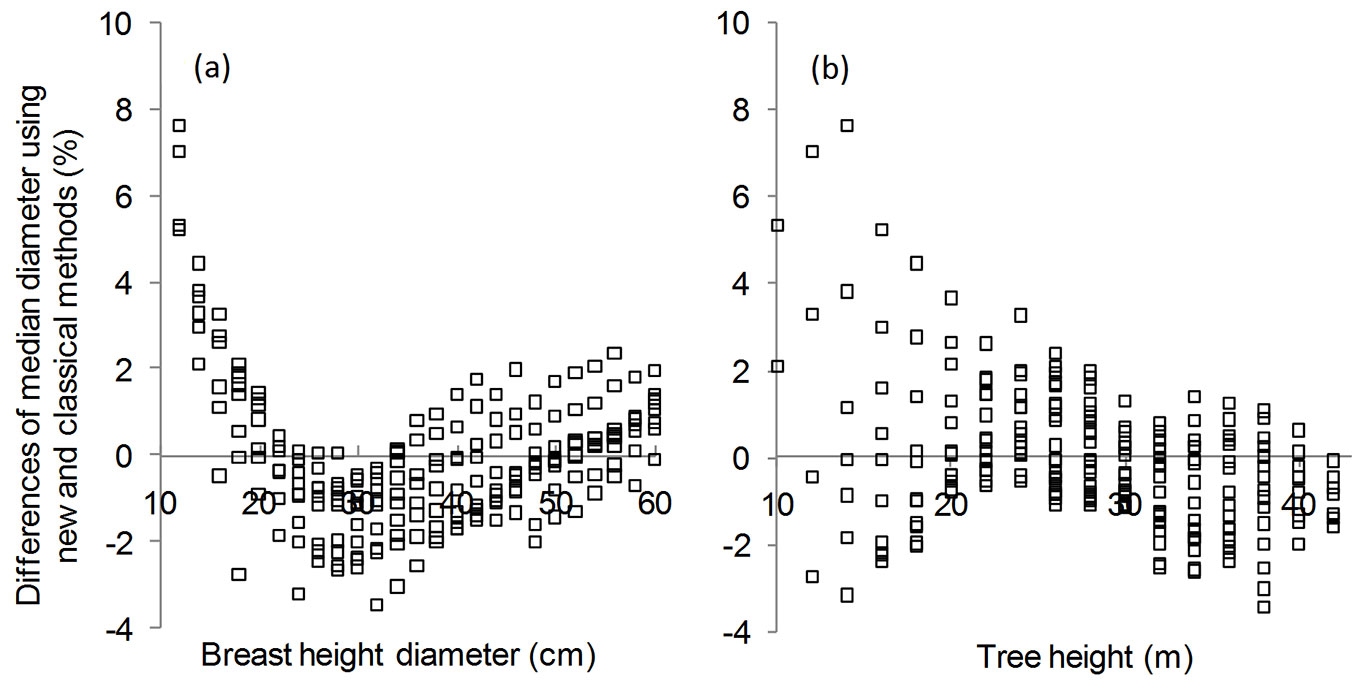
We also calculated the relative deviation of median diameter estimates obtained with the two aforementioned methods using both DBH and H as predictors (Fig. 5a, Fig. 5b). Results showed that such deviation was lower than 2% in 86.24% of the analyzed trees. The observed relative differences amounted to 7.60% for smaller trees in the range 12-14 cm for DBH, or 10-14 m for H. Similar differences were obtained when comparing diameters measured at 0.3 × H to the median diameter of the stem profile using the classical method.
Fig. 5 - Relative difference (%) of median diameter estimates with: (a) the class of breast height diameter; (b) the tree height class. The depicted model for predicting median diameter values is: DM = 0.7061 DBH + 3.235.
Tab. 1 displays the best fitted models for the median diameter of the stem profile, and absolute and relative height of the median diameter position. As expected, DM, HDM, and HDMrel were best predicted by using both independent variables (DBH and H) as predictors.
Tab. 1 - Predictive models of median diameter and its absolute and relative height along the stem obtained by multiple regression analysis.
| Best fitted models | R 2 | df | F | P |
|---|---|---|---|---|
| DM = 2.716099 + 0.688689 DBH + 0.041612 H | 0.999055 | 2, 215 | 113689.80 | < 0.0001 |
| HDM = -0.703391 - 0.029121 DBH + 0.365176 H | 0.998636 | 2, 215 | 78681.77 | < 0.0001 |
| HDMrel = 0.264829 - 0.000983 DBH + 0.002511 H | 0.873587 | 2, 215 | 742.88 | < 0.0001 |
Model selection for practice
Our results indicated that two aforementioned methods could be used to obtain a rapid assessment of median diameter of the stem profile for Norway spruce trees in Romania, specifically: (i) using eqn. 6 based on tree DBH, or its simplified form to be applied when less accuracy is required (eqn. 12):
(ii) measuring the stem diameter at 0.3 × H distance from the base of the trunk.
The first method only involves measuring breast height diameter and a simple calculation for both standing and felled trees. The second method requires the measurement of tree length (considered to be equal to tree height), followed by the direct measurement of the median diameter at 0.3 × H distance from the stem base. These results support the empirical evidence of Forslund ([10]), whereby the average center of gravity of aspen boles without branches is located at 3/10 of the bole height from its base. Wood et al. ([41]) also showed the importance of the point on the stem at a relative height of approximately 0.3. They obtained a minimum bias in the estimation of tree volume, using centroid sampling for 114 trees of radiata pine, where the point of sampling was fixed at the height of the centroid.
Perspectives of easier computation of the median diameter
The practical implications of the proposed methods are relevant, particularly when the relative height of the median diameter is correlated with the distribution of volume in trees. These methods should be applied to other species to validate their use, especially in the case of conifers. The Mathiesen’s formula for volume estimation could be modified and improved by using the proposed models. Our results demonstrated the feasibility of assessing the stand volume using the median diameter of the stem profile. For two species of pines (loblolly and ponderosa pine), Coble & Wiant ([6]) illustrated the accuracy of the centroid method for determining volumes of standing trees, requiring only the diameter measurement of one upper stem.
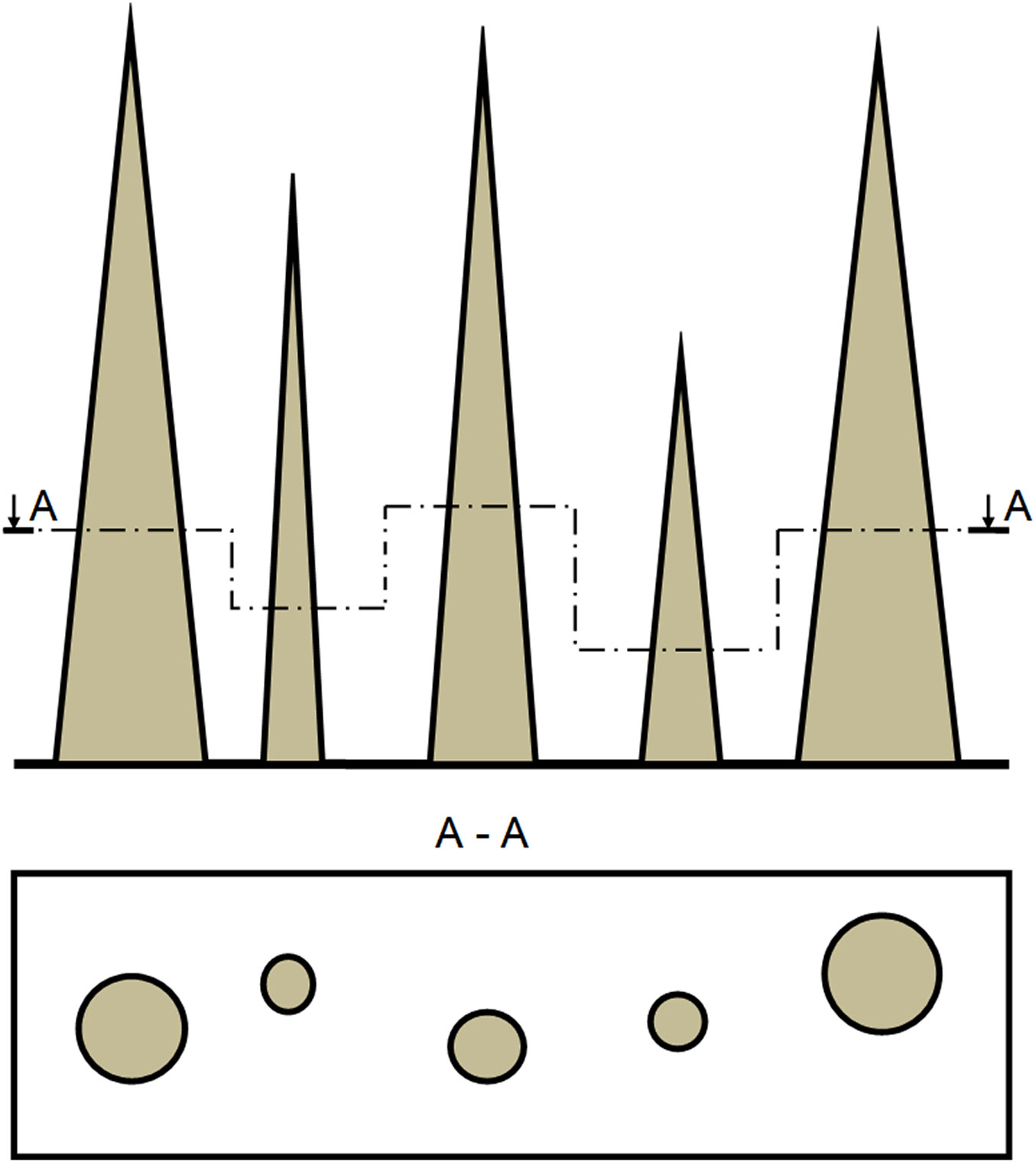
The median diameter of the stem profile could be used to study stand architecture and competition between trees (Fig. 6). Indeed, median diameter distributions from forest inventories provide a more precise way of describing forest stand structure and the relationships between trees. Moreover, DM may allow the development of forest growth models (similar to those used in Switzerland) to estimate the growth of single trees based on DBH, H, and a given upper stem diameter ([36]). Furthermore, the possibility of using the 0.3 × H estimated in this study as an index of tree competition could be explored. Biging & Dobbertin ([4]) proposed several crown competition indexes based on evaluation at one-third, one-half, two-thirds and other relative heights of study trees.
Fig. 6 - Schematic representation of the stand structure using the median diameter of stem profile as a descriptor. The horizontal projection of the cross-sectional area at the height of the median diameter of trees could be considered an alternative approach to study tree growth, tree resistance to wind, and forest dynamics.
Compared to DBH, DM provides a more accurate prediction of the stem form. It also provides a better way of indicating the response of tree form to variability in environmental factors. For instance, Pollanschütz ([26]) used a three-variate (DBH, H, and diameter at 0.3 × H) model to calculate the form factor. This point along the stem better characterizes the ability of a tree to respond to ecological factors. Fifty percent of the area of the stem profile and approximately 50% of the stem volume are concentrated below the cross-sectional area at the height of median diameter (0.3 × H, according to our study). Moreover, the horizontal projection of the cross-sectional area corresponding to DM might represent a new approach to assess tree stability and failure. Furthermore, the core of juvenile wood below the crown is more or less constant, whereas that of adult wood covering this core decreases from the base to the tip ([5]). Therefore, the cross section of the stem located near the median diameter exhibits a particular mechanical behavior. Indeed, Young’s modulus seems to be at its lowest in the bottom part of Norway spruce trees ([5]).
Resistance of a tree depend on many factors. Gardiner et al. ([12]) observed that stem characteristics (diameter and wood strength), root plate morphology, soil type, and soil moisture vary widely depending on the location of the tree crown within the canopy, tree species, stand location, and wind exposure. A tree is assumed to break when the stress acting on the stem exceeds a critical value of the modulus of rupture ([24]). Based on this assumption, Peltola et al. ([24]) developed a model to assess the risk of wind and snow damage to single trees and stands of Norway spruce. For individual Scots pine and Norway spruce, Gardiner et al. ([11]) compared two models developed to predict the critical wind speed required to damage coniferous trees with varying tree height and stem taper (DBH/H). The ratio defined by the median diameter of the stem profile and tree height (DM/H) helps to determine the breaking strength of the stem and, hence, tree resistance to overturning. Ancelin et al. ([2]) also developed a model of tree response to wind, based on the numerical description of tree structure. This concept is compatible with a model of coniferous forest stand dynamics. Thus, we recommend using the median diameter of the stem profile to predict the sensitivity of trees breakage and bending.
Conclusions
Our study developed two methods to assess the median diameter of the stem profile without measuring multiple diameters at different heights along the stem. The median diameter may be obtained without classical computations that require the area of the stem profile. We demonstrated that diameter at 0.3 × height is a good approximation of the median diameter. Accuracy analysis of the proposed models indicates differences < 2% in most cases. Tree distribution based on the median diameter of the stem profile represents a new direction for forest dynamics studies. The ratio between the median diameter of stem profile and the tree height (DM/H) could be applied for measuring other economically important factors, including the breaking strength of the stem and tree resistance to overturning.
Acknowledgements
We thank Andres Kiviste and Allan Sims (Institute of Forestry and Rural Engineering, Estonian University of Life Science) for providing Mathiesen’s original paper (1925). We thank Paolo Cherubini (WSL Swiss Federal Institute for Forest, Snow and Landscape Research), Tudor Stancioiu, and Alexandru Borz (Faculty of Silviculture and Forest Engineering, Transilvania University of Brasov) for providing valuable comments on the manuscript.
Author contributions
MMV originally formulated the concept and elaborated the database; MMV and CCT developed the methodology; MMV, FD, and CCT analysed the data; MMV formulated the mathematical models; and MMV and FD wrote the manuscript.
References
Gscholar
Gscholar
Gscholar
Gscholar
Gscholar
Gscholar
Gscholar
Gscholar
Gscholar
Gscholar
Gscholar
Gscholar
Gscholar
Gscholar
Gscholar
Gscholar
Gscholar
Supplementary Material
Authors’ Info
Authors’ Affiliation
Cornel Cristian Teresneu
Florin Dinulica
Department of Forest Engineering, Forest Management Planning and Terrestrial Measurements, Faculty of Silviculture and Forest Engineering, Transilvania University of Brasov, Sirul Beethoven 1, 500123, Brasov (Romania)
Corresponding author
Paper Info
Citation
Vasilescu MM, Teresneu CC, Dinulica F (2017). A rapid method for estimating the median diameter of the stem profile of Norway spruce (Picea abies Karst) trees. iForest 10: 328-333. - doi: 10.3832/ifor1945-009
Academic Editor
Emanuele Lingua
Paper history
Received: Dec 12, 2015
Accepted: Sep 07, 2016
First online: Feb 11, 2017
Publication Date: Feb 28, 2017
Publication Time: 5.23 months
Copyright Information
© SISEF - The Italian Society of Silviculture and Forest Ecology 2017
Open Access
This article is distributed under the terms of the Creative Commons Attribution-Non Commercial 4.0 International (https://creativecommons.org/licenses/by-nc/4.0/), which permits unrestricted use, distribution, and reproduction in any medium, provided you give appropriate credit to the original author(s) and the source, provide a link to the Creative Commons license, and indicate if changes were made.
Web Metrics
Breakdown by View Type
Article Usage
Total Article Views: 47368
(from publication date up to now)
Breakdown by View Type
HTML Page Views: 40017
Abstract Page Views: 2439
PDF Downloads: 3864
Citation/Reference Downloads: 19
XML Downloads: 1029
Web Metrics
Days since publication: 3141
Overall contacts: 47368
Avg. contacts per week: 105.56
Article Citations
Article citations are based on data periodically collected from the Clarivate Web of Science web site
(last update: Mar 2025)
Total number of cites (since 2017): 4
Average cites per year: 0.44
Publication Metrics
by Dimensions ©
Articles citing this article
List of the papers citing this article based on CrossRef Cited-by.
Related Contents
iForest Similar Articles
Research Articles
Allometric relationships for predicting the stem volume in a Dalbergia sissoo Roxb. plantation in Bangladesh
vol. 3, pp. 153-158 (online: 15 November 2010)
Research Articles
Comparative analysis of taper models for Pinus nigra Arn. using terrestrial laser scanner acquired data
vol. 17, pp. 203-212 (online: 22 July 2024)
Research Articles
Allometric models for the estimation of foliage area and biomass from stem metrics in black locust
vol. 15, pp. 281-288 (online: 27 July 2022)
Research Articles
Prediction of stem diameter and biomass at individual tree crown level with advanced machine learning techniques
vol. 12, pp. 323-329 (online: 14 June 2019)
Research Articles
Estimation of stand crown cover using a generalized crown diameter model: application for the analysis of Portuguese cork oak stands stocking evolution
vol. 9, pp. 437-444 (online: 02 December 2015)
Short Communications
Climate effects on growth differ according to height and diameter along the stem in Pinus pinaster Ait.
vol. 11, pp. 237-242 (online: 12 March 2018)
Research Articles
Simplified methods to inventory the current annual increment of forest standing volume
vol. 5, pp. 276-282 (online: 17 December 2012)
Research Articles
Estimating the mechanical stability of Pinus nigra Arn. using an alternative approach across several plantations in central Italy
vol. 8, pp. 846-852 (online: 08 April 2015)
Review Papers
Integration of forest mapping and inventory to support forest management
vol. 3, pp. 59-64 (online: 17 May 2010)
Research Articles
Modeling compatible taper and stem volume of pure Scots pine stands in Northeastern Turkey
vol. 16, pp. 38-46 (online: 22 January 2023)
iForest Database Search
Search By Author
Search By Keyword
Google Scholar Search
Citing Articles
Search By Author
Search By Keywords
PubMed Search
Search By Author
Search By Keyword