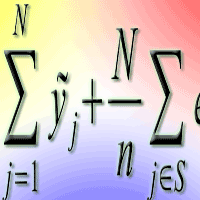Site quality assessment of degraded Quercus frainetto stands in central Greece

doi:10.3832/ifor1069-007

Site quality assessment of degraded Quercus frainetto stands in central Greece

Kyriaki Kitikidou⁽¹⁾ , Elias Milios⁽¹⁾, Elias Tsirekis⁽¹⁾, Elias Pipinis⁽²⁾, Athanasios Stampoulidis⁽¹⁾

iForest - Biogeosciences and Forestry, Volume 8, Issue 1, Pages 53-58 (2015)
doi: https://doi.org/10.3832/ifor1069-007
Published: May 12, 2014 - Copyright © 2015 SISEF

Research Articles

PDF Version

Full Text

Citation

Geo-mapping

The potential yield of a site is measured by site index, which is defined as the dominant height of a stand at a base age. A site index model for site quality assessment of Quercus frainetto (Hungarian oak) stands in central Greece was developed using a base age of 50 years. Data were collected from 39 temporary sample plots of 10 x 10 m. Linear regression models widely used in site index studies were fitted to height-age data. The adjusted coefficient of determination (R²_adj), root mean square error (RMSE), bias, coefficient of determination for the prediction (R²_pr) and residual plots were used for the choice of the best-fitting model. The best model was H = -0.231+0.251A-0.001A², where H is the predicted height at age A. The guide curve method was adopted in constructing the sites curves, with the chosen model as the guide curve. Based on this curve, the study area was divided into three site quality classes (I to III), with class I representing the best and class III the poorest. Also, the presence of a Simpson’s paradox in these analyses is discussed. The results showed that a 50-year-old stand in the study area attained an average dominant height of about 11, 8 and 6 m on site quality classes I, II and III, respectively. The Hungarian oak stands of the present study can be considered very low productivity stands.

Keywords

Guide Curve Method, Hungarian Oak, Simpson’s Paradox, Site Quality

Authors’ address

(1)

Department of Forestry and Management of the Environment and Natural Resources, Democritus University of Thrace, Pandazidou 193, GR-68200 Orestiada (Greece)

(2)

Laboratory of Silviculture, Faculty of Forestry and Natural Environment, Aristotle University of Thessaloniki, GR-54124 Thessaloniki (Greece)

Corresponding author

Kyriaki Kitikidou
kkitikid@fmenr.duth.gr

Citation

Kitikidou K, Milios E, Tsirekis E, Pipinis E, Stampoulidis A (2015). Site quality assessment of degraded Quercus frainetto stands in central Greece. iForest 8: 53-58. - doi: 10.3832/ifor1069-007

Academic Editor

Raffaele Lafortezza

Paper history

Received: Jul 11, 2013
Accepted: Jan 26, 2014

First online: May 12, 2014
Publication Date: Feb 02, 2015
Publication Time: 3.53 months

Open Access

This article is distributed under the terms of the Creative Commons Attribution-Non Commercial 4.0 International (https://creativecommons.org/licenses/by-nc/4.0/), which permits unrestricted use, distribution, and reproduction in any medium, provided you give appropriate credit to the original author(s) and the source, provide a link to the Creative Commons license, and indicate if changes were made.

Breakdown by View Type

(Waiting for server response...)

Article Usage

Total Article Views: 48056
(from publication date up to now)

Breakdown by View Type
HTML Page Views: 40882
Abstract Page Views: 2587
PDF Downloads: 3518
Citation/Reference Downloads: 28
XML Downloads: 1041

Web Metrics
Days since publication: 3848
Overall contacts: 48056
Avg. contacts per week: 87.42

Article Citations

Article citations are based on data periodically collected from the Clarivate Web of Science web site
(last update: Feb 2023)

Total number of cites (since 2015): 6
Average cites per year: 0.67

(no Plumx Metrics available for this paper)

Publication Metrics

by Dimensions ^©

Articles citing this article

List of the papers citing this article based on CrossRef Cited-by.

(1)

Abramson N, Kelsey S, Safar P, Sutton-Tyrell K (1992)
Simpson’s paradox and clinical trials: what you find is not necessarily what you prove. Annals of Emergency Medicine 21: 1480-1482.
CrossRef | Gscholar

(2)

Alder D (1980)
Forest volume estimation and yield prediction. Volume 2 - Yield prediction. FAO Forestry Paper 22/2, FAO, Rome, Italy, pp. 194.
Gscholar

(3)

Beekhuis J (1966)
Prediction of yield and increment in Pinus radiata stands in New Zealand. Technical Paper No 49, New Zealand Forest Research Institute, Rotorua, New Zealand, pp. 40.
Gscholar

(4)

Bergmeier E, Dimopoulos P (2008)
Identifying plant communities of thermophilous deciduous forest in Greece: species composition, distribution, ecology and syntaxonomy. Plant Biosystems 142 (2): 228-254.
CrossRef | Gscholar

(5)

Carmean W (1972)
Site index curves for upland oaks in the Central States. Forest Science 18: 109-120.
Online | Gscholar

(6)

Christensen KI (1997)
Fagaceae. In: “Flora Hellenica” (Strid A, Tan K eds). Koeltz Scientific Books, Koenigstein, Germany, pp. 40-50.
Gscholar

(7)

Clutter J, Fortson J, Piennar L, Brister G, Bailey R (1983)
Timber management: a quantitative approach. John Wiley and Sons, New York, USA, pp. 333.
Gscholar

(8)

Cohen J (1986)
An uncertainty principle in demography and the unisex issue. The American Statistician 40 (1): 32-39.
Online | Gscholar

(9)

Curtis R, Demars D, Herman F (1974)
Which dependent variable in site index height-age regression? Forest Science 20: 74-87.
Online | Gscholar

(10)

Dafis S (1966)
Site and yield researches in coppice oak and chestnut forests of North-Eastern Chalkidiki (Northern Greece). Internal Report, Department of Forestry and Natural Resources, Aristotle University of Thessaloniki, Thessaloniki, Greece.
Gscholar

(11)

Gadow K, Hui G (1998)
Modeling forest development. Kluwer Academic Publishers, Dordrecht, The Netherlands, pp. 213.
Online | Gscholar

(12)

Loh W (2006)
Logistic regression tree analysis. In: “Handbook of Engineering Statistics” (Pham H ed). Springer, pp. 537-549.
CrossRef | Gscholar

(13)

Makridakis S, Wheelwright S, Hyndman R (1998)
Forecasting methods and applications (3rd edn). John Wiley & Sons, New York, USA, pp. 642.
Gscholar

(14)

Matis K (2000)
Height growth and site index curves for Quercus conferta Kit. in the University forest at Taxiarchis, Greece. Geotechnical Scientific Issues 11 (2): 64-76.
Gscholar

(15)

Ministry of Agriculture (1992)
Results of the first National Forest Inventory of Greece. General Secretariat of Forests and Natural Environment, Athens, Greece, pp. 134.
Gscholar

(16)

Myers R (1986)
Classical and modern regression with applications. Duxbury Press, Boston, MS, USA, pp. 488.
Gscholar

(17)

Nanang D, Nunifum T (1999)
Selecting a functional form for anamorphic site index curve estimation. Forest Ecology and Management 118: 211-221.
CrossRef | Gscholar

(18)

Newberry J (1991)
A note on Carmean’s estimate of height from stem analysis data. Forest Science 37 (1): 368-369.
Online | Gscholar

(19)

Piñeiro G, Oesterheld M, Batista W, Paruelo J (2006)
Opposite changes of whole-soil vs. pools C:N ratios: a case of Simpson’s paradox with implications on nitrogen cycling. Global Change Biology 12: 804-809.
CrossRef | Gscholar

(20)

Rinn F (2003)
TSAP-Win user reference manual. RinnTech, Heidelgerg, Germany, pp. 91.
Gscholar

(21)

Scheiner S, Cox S, Willig M, Mittelbach G, Osenber C, Kaspari M (2000)
Species richness, species-area curves and Simpson’s paradox. Evolutionary Ecology Research 2: 791-802.
Gscholar

(22)

Smiris P, Aslanidou M, Milios E (1998)
Oak (Quercus conferta Kit.) thinnings at Cholomonda, Chalkidiki (Northern Greece). In: Proceedings of the “8th Pan-Hellenic Conference of the Greek Forestry Society”. Alexandroupoli (Greece) 6-8 Apr 1998. Greek Forestry Society, Tessaloniki, Greece, pp. 417-424.
Gscholar

(23)

Smiris P, Ganatsas P, Efthymiou G (1992)
Forest dynamics of two oak stands under restoration. Scientific Annals of the Department of Forestry and Natural Resources, Aristotle University of Thessaloniki, Greece, vol. 35/2, pp. 607-626.
Gscholar

(24)

Soares P, Tomé M, Skovsgaard J, Vanclay J (1995)
Evaluating a growth model for forest management using continuous forest inventory data. Forest Ecology and Management 71: 251-265.
CrossRef | Gscholar

(25)

Teshome T, Petty J (2000)
Site index equation for Cupressus lusitanica stands in Munessa, Ethiopia. Forest Ecology and Management 126: 339-347.
CrossRef | Gscholar

(26)

Thomas CH, Parresol R (1989)
Comparing basal area growth rates in repeated inventories: Simpson’s paradox in forestry. Forest Science 35 (4): 1029-1039.
Gscholar

(27)

Trousdell K, Beck D, Lioyd F (1974)
Site index for loblolly pine in the Atlantic Central Plain of the Carolina and Virginia. Research Paper SE-115, USDA Forest Service, USA, pp. 11.
Gscholar

(28)

Vanclay J (1994)
Modeling forest growth and yield: applications to mixed tropical forests. CABI Publishing, Wallingford, UK, pp. 336.
Gscholar

(29)

Wagner C (1998)
Simpson’s paradox in real life. American Statistician 36: 46-48.
Gscholar

(30)

Walters D, Gregoire T, Burkhart H (1989)
Consistent estimation of site index curves fitted to temporary plot data. Biometrics 45 (1): 24-33.
CrossRef | Gscholar

(31)

Wang Y, Payandeh B (1995)
A base-age invariant site index model for aspen stands in north central Ontario. Forest Ecology and Management 72: 207-211.
CrossRef | Gscholar

(32)

Xirogiannis A (2001)
Management plan of state oak forests at North-Western Vardousia mountains (central Greece), for the period 2000-2009. Forest Service, Sperchiada, Greece, pp. 322.
Gscholar

iForest - Biogeosciences and Forestry

Contents

Search

Journal Info

Journal Subjects and Fields

For Authors

For Reviewers

For Readers

SISEF Publishing

Search iForest Contents