Dynamic modelling of target loads of acidifying deposition for forest ecosystems in Flanders (Belgium)
iForest - Biogeosciences and Forestry, Volume 2, Issue 1, Pages 30-33 (2009)
doi: https://doi.org/10.3832/ifor0484-002
Published: Jan 21, 2009 - Copyright © 2009 SISEF
Short Communications
Collection/Special Issue: Cost Action E29 Meeting 2008 - Istanbul (Turkey)
Future Monitoring and Research Needs for Forest Ecosystems
Guest Editors: Marcus Schaub (WSL, Birmensdorf, CH)
Abstract
The aim of this study was to determine target loads for acidification for representative forest ecosystems in Flanders (Belgium) using dynamic modelling. Target loads were calculated for 83 forest stands for which sufficient measurements were available. This dataset is considered to be representative for the Flemish forest area. It is concluded that, despite the inherent uncertainty in modelling soil acidification at a regional level, important N and S deposition reductions are needed to allow recovery of the Flemish forest soils.
Keywords
Acidifying deposition, Critical load, Ecosystem recovery, VSD model, Target load
Introduction
The input of nitrogen (N) and sulphur (S) to terrestrial and aquatic ecosystems has strongly increased over the past century due to human activities. This particularly holds true for forest ecosystems in Europe ([3]) and NE America ([4]). Chronically enhanced N and S deposition results in varying ecosystem responses, including increased biomass growth, but also soil acidification, changes in plant species composition and nutrient availability, increased N emission losses, and increased nitrate, sulphate, and cation losses by leaching below the rooting zone ([1], [13]).
For forest and nature policy it is important to know the highest deposition level below which no harmful effects on forest soils occur. Therefore, the concept of critical loads has been adopted, i.e., the highest deposition of acidifying compounds that will not cause chemical changes leading to long-term harmful effects on ecosystem structure and function according to present knowledge ([9]). Critical loads are determined using a static mass balance in which the ecosystem is assumed to be in equilibrium with the deposition. However, many forest ecosystems are not in steady-state due to processes such as cation exchange, sulphate desorption and N immobilisation, which create time lags between changes in deposition and chemical soil status (e.g., [5]). Consequently, dynamic models that account for these buffering processes are required to simulate the effect of changing atmospheric inputs on forest soils through time. This allows to determine a target load, i.e., the deposition for which a chosen chemical status is respected from a target year on ([9]).
The N and S deposition in Flanders (northern Belgium) is amongst the highest in Europe ([10]). While critical loads have been calculated for the acidifying and eutrophying effects of N and S deposition onto various ecosystems, soil buffering mechanisms have not yet been taken into account. Therefore, the aim of this study was to determine target loads for acidification for representative forest ecosystems in Flanders using dynamic modelling.
Summary of methods
Critical loads and target loads were calculated for 83 forest stands of the Flemish forest condition (Level I) and intensive monitoring (Level II) networks according to the harmonized methodology of the Coordination Center of Effects ([9]). The Very Simple Dynamic (VSD) model was used for simulating the chemical composition of soil and soil solution throughout time (1880-2100) based on soil characteristics and rainfall, deposition and growth data. The VSD model is largely based on the soil model SMART ([2], [6]) that has been simplified by: (i) neglecting calcareous soils and aluminium depletion in highly acidified soils; (ii) ignoring sulphate adsorption; (iii) assuming complete nitrification, thus ignoring NH4 + leaching; and (iv) lumping the exchange of all base cations in one term. Furthermore, it uses a new approach of modelling N immobilisation. More information on VSD is given in Reinds et al. ([7]). In the present study, the mass balance of N included atmospheric deposition, soil immobilisation, growth uptake, denitrification, and soil leaching. The exact VSD equations used are given in UBA ([9]). The year 2010 was used as the starting year of deposition reductions, with 2020 the implementation year of a target load. Target loads were calculated that resulted in a maximum aluminium to base cation ratio (molar Al:Bc = 1) in the soil solution from three target years on (2030, 2050, and 2100).
Input data were as much as possible derived from site-specific measurements or regional data, following guidelines of UBA ([9]) and described in detail by Staelens et al. ([8]). Soil parameters included (i) mineral weathering of base cations (K+, Ca2+, and Mg2+), estimated from the measured soil type, (ii) cation exchange, derived from the measured cation exchange capacity and the Gapon exchange model, with exchange coefficients calibrated by the VSD model, (iii) denitrification, estimated from soil drainage status and assuming total nitrification, and (iv) nitrogen immobilisation, modelled based on the measured carbon to nitrogen (C:N) ratio of the litter and upper 0-20 cm soil layers. Time series of atmospheric N and S deposition were provided by EMEP (Convention on Long-range Transboundary Air Pollution, CLRTAP). Base cation and chloride deposition were assumed to be constant over time and were derived from throughfall data corrected for ion exchange within the canopy. The growth uptake of nitrogen and base cations was calculated using tree species-specific annual wood production and nutrient contents. The annual percolation flux below the rooting zone was quantified by combining regionally interpolated rainfall with species-specific rainfall interception and actual evapotranspiration values. Model sensitivity was assessed for all stands by varying the input data and model parameters within reasonable ranges.
Critical loads and target loads of acidifying deposition indicate a sum of N and S deposition that allow the soil and soil solution to reach a chemical criterion in the long term or in the target year, respectively. If only S contributes to soil acidification, the acceptable S deposition is called the maximum critical or target load of S. If the S deposition is zero, the acceptable N deposition is the maximum critical or target load of acidifying N ([9]).
Results and discussion
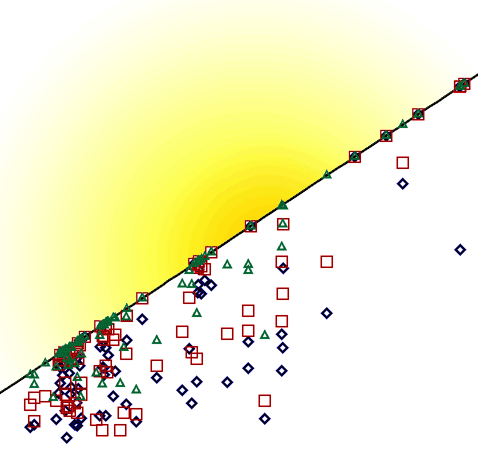
Target loads depend on the target year by which the preset chemical criterion (Al:Bc = 1) in the soil solution should be achieved at last. Target loads are higher for more future target years (Fig. 1) as this corresponds to allowing a longer time period with lowered N and S deposition during which soils can recover from acidification. To respect the Al:Bc criterion from target year 2050 on, stronger reductions in N and S deposition than in the Gothenburg agreements (CLRTAP) were needed in 84% of the plots. In 12% of the plots no additional deposition reductions were needed, while for 4% the criterion could not be reached. For target year 2030, the criterion was not feasible for 17% of the plots.
Fig. 1 - Relationship between target loads for 2030, 2050, and 2100 and critical loads for (A) sulphur, S, and (B) acidifying nitrogen, N, for 83 Flemish forest sites. Note: the diagonal indicates a 1:1 ratio.
The median (n = 83) target load of S for 2030, 2050, and 2100 amounted to 58, 65, and 86% of the median critical load of S (being 1829 eq ha-1 yr-1), respectively (Tab. 1). The target loads of N differed less from the critical load of N than for S due to the time-dependent soil N immobilisation. Target and critical loads of N were always higher than for S because of this N immobilisation, N growth uptake and denitrification. However, enhanced N inputs not only affect terrestrial ecosystems through soil acidification (cf. Introduction). To avoid changes in species composition or nitrate leaching to the groundwater, other critical loads are used ([9]) that result in lower acceptable N loads than in the present study ([8]), in which we calculated the acceptable acidifying N deposition.
Tab. 1 - 5th, 50th (median), and 95th percentile values of the target load for three target years and critical load (eq ha-1 yr-1) for sulphur (S) and acidifying nitrogen (N) for 83 Flemish forest sites.
| Loads | S (eq ha-1 yr-1) | N (eq ha-1 yr-1) | ||||
|---|---|---|---|---|---|---|
| 5% | 50% | 95% | 5% | 50% | 95% | |
| Target load 2030 | 0 | 811 | 2464 | 0 | 1938 | 7947 |
| Target load 2050 | 41 | 1324 | 2873 | 747 | 2564 | 8011 |
| Target load 2100 | 478 | 1598 | 2885 | 1691 | 2958 | 8064 |
| Critical load | 919 | 1829 | 2885 | 2162 | 3115 | 8374 |
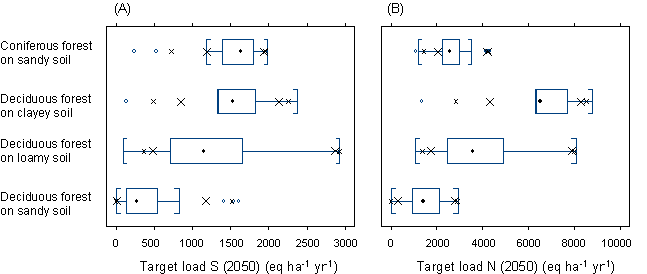
The critical and target loads were lower for deciduous than for coniferous stands (Fig. 2) because of the higher base cation uptake by deciduous trees. As an example, for sandy soils the median target load of S for 2050 was 275 eq ha-1 yr-1 for deciduous stands (n = 25) and 1638 eq ha-1 yr-1 for coniferous stands (n = 27). The acceptable acidifying deposition was lowest for forests on sandy soils because of the lower mineral weathering rates compared to loamy or clayey soils (Fig. 2 - [11]).
Fig. 2 - Boxplots (n = 83) of target loads for (A) sulphur, S, and (B) acidifying nitrogen, N, for target year 2050 for the main combinations of forest type and soil type in Flanders. Note: The closed rectangle of the boxplots indicates the interquartile range (25-75%) of the distribution, the dot being the median. The small x and large X left (right) of the rectangle show the 5th (95th) and 10th (90th) percentiles, respectively. The [ ] range comprises all values closer to the median than 1.5 times the interquartile range. Values outside the [ ] range are shown individually as open circles.
According to the sensitivity analysis (Tab. 2), the calculated target loads for 2050 depended mostly on the base cation fluxes by mineral weathering, deposition, and net growth uptake. Furthermore, the results were clearly affected by the chosen cation exchange model (Gapon vs. Gaines-Thomas) and the assumed relationship between the soil solution pH and aluminium concentration (cf. [12]). For many variables, target loads were more sensitive to changes than critical loads. A 20% change in the atmospheric deposition of base cations affected the target loads of S more than twice as much as the critical loads. This can be explained by the fact that in the VSD model a constant base cation deposition over the entire simulation period is assumed.
Tab. 2 - Median (n = 83) target load (TL, target year 2050) and critical load (CL) for sulphur (S) and acidifying nitrogen (N) using modified input parameters and variables. All values are expressed as the difference (%) compared to the reference approach values (eq ha-1 yr-1). (-): Parameter not used for calculating critical loads.
| Input parameter | Modification | Median TL 2050 | Median CL | ||
|---|---|---|---|---|---|
| S | N | S | N | ||
| Reference approach | 1324 | 2564 | 1829 | 3115 | |
| Mineral weathering rate (BCw) | BCw + 20% | 14.5 | 9.4 | 3.2 | 3.3 |
| BCw - 20% | -21.7 | -10.5 | -12.9 | -4.9 | |
| Denitrification factor (fde) | fde + 20% | 2.7 | 9.4 | 0.0 | 14.9 |
| fde - 20% | -3.1 | -7.6 | 0.0 | -9.3 | |
| Minimal denitrification | fde = 0.1 | -13.2 | -15.0 | 0.0 | -19.6 |
| Acceptable N immobilisation (Ni,acc) | Ni,acc + 20% | 0.4 | 2.4 | 0.0 | 1.9 |
| Ni,acc - 20% | 0.0 | -2.0 | 0.0 | -1.9 | |
| Minimal N immobilisation | Ni,acc = 1 kg ha-1 yr-1 | -0.9 | -8.2 | 0.0 | -5.2 |
| Deposition of base cations (Bcdep) | Bcdep + 20% | 48.5 | 38.5 | 23.6 | 25.7 |
| Bcdep - 20% | -56.2 | -33.4 | -24.6 | -24.3 | |
| Growth uptake of nitrogen (Nu) and base cations (Bcu) |
Nu and Bcu + 20% | -22.4 | -10.4 | -14.1 | -7.2 |
| Nu and Bcu - 20% | 17.1 | 12.0 | 5.3 | 9.2 | |
| Bcu + 20% | -23.2 | -14.8 | -14.1 | -9.2 | |
| Bcu - 20% | 20.7 | 17.6 | 5.3 | 11.3 | |
| Nu + 20% | 1.3 | 4.8 | 0.0 | 3.3 | |
| Nu - 20% | -1.3 | -7.3 | 0.0 | -3.3 | |
| Drainage flux (Q) | Q + 20% | -1.8 | -0.3 | -0.3 | -0.5 |
| Q - 20% | 1.3 | -0.2 | 0.2 | 0.4 | |
| Parameters for Al3+-H+ concentration relationship (logKAlox, expAl) |
logKAlox + 0.5 | -9.0 | -5.1 | -4.6 | -4.2 |
| logKAlox - 0.5 | 10.6 | 5.4 | 5.9 | 5.8 | |
| Dutch empirical values | ([9]) | -11.9 | 10.4 | 13.0 | 23.0 |
| Dissociation of organic anions Concentration (m·DOC) |
m·DOC + 20% | -4.9 | -1.1 | -1.7 | -1.7 |
| mDOC - 20% | 4.5 | 1.1 | 1.6 | 1.7 | |
| mDOC = 0 | 14.1 | 11.7 | 7.4 | 8.5 | |
| Fixed dissociation constant (pK1) Factor partialCO2 pressure (pCO2) |
pK1 = 4.5 | 7.7 | 2.7 | 3.7 | 3.8 |
| pCO2 = 25 | -0.4 | -0.1 | -0.2 | -0.2 | |
| pCO2 = 5 | 0.9 | 0.1 | 0.2 | 0.2 | |
| Cation exchange capacity (CEC) | CEC + 20% | -8.3 | -4.3 | - | - |
| CEC - 20% | 5.9 | 5.7 | - | - | |
| Base saturation (BS) | BS + 20% | -6.9 | -2.1 | - | - |
| BS - 20% | 3.7 | 6.0 | - | - | |
| Cation exchange model | Gaines-Thomas | -31.8 | -25.7 | - | - |
| N deposition (Ndep) | Ndep + 20% | -2.7 | -3.0 | - | - |
| Ndep - 20% | 2.9 | 5.2 | - | - | |
| S deposition (Sdep) | Sdep + 20% | -21.1 | -7.5 | - | - |
| Sdep - 20% | 11.9 | 7.8 | - | - | |
| N and S deposition | Ndep and Sdep + 20% | -23.8 | -8.0 | - | - |
| Ndep and Sdep - 20% | 15.9 | 11.5 | - | - | |
Exceedances of target loads were calculated based on projected EMEP depositions for 2010. For target year 2050, the projected depositions were too high for 88% of the study sites. The median exceedances of acidifying N and S deposition for the target years 2030, 2050, and 2100 were 1953, 1443, and 1202 eq ha-1 yr-1.
In the present study, target loads were calculated for 83 forest stands for which sufficient measurements were available. Thanks to the systematic site selection of the forest condition network, this dataset is considered to be representative for the Flemish forest area in general, although coniferous stands were slightly under-represented. This was confirmed by comparing the distribution of critical loads for acidification for the 83 sites with those for a larger database of 1438 plots ([8]). The range of critical S loads was similar for both datasets, and the median values differed by only 6%. We conclude that, despite the inherent uncertainty in modelling soil acidification at a regional level, important N and S deposition reductions are needed to allow recovery of the Flemish forest soils.
Acknowledgements
This study was funded by the Flemish Environment Agency (VMM - MIRA) and implemented at the Research Institute for Nature and Forest (INBO). The first author is currently granted as a post-doctoral fellowship at Ghent University by the Research Foundation Flanders (FWO-Vlaanderen).
References
Gscholar
Gscholar
Gscholar
Authors’ Info
Authors’ Affiliation
Laboratory of Forestry, Laboratory of Applied Physical Chemistry, Ghent University, Coupure links 653, 9000 Gent (Belgium)
J Neirynck
G Genouw
P Roskams
Research Institute for Nature and Forest, Gaverstraat 4, 9500 Geraardsbergen (Belgium)
Corresponding author
Paper Info
Citation
Staelens J, Neirynck J, Genouw G, Roskams P (2009). Dynamic modelling of target loads of acidifying deposition for forest ecosystems in Flanders (Belgium). iForest 2: 30-33. - doi: 10.3832/ifor0484-002
Academic Editor
Marcus Schaub
Paper history
Received: Mar 13, 2008
Accepted: Dec 09, 2008
First online: Jan 21, 2009
Publication Date: Jan 21, 2009
Publication Time: 1.43 months
Copyright Information
© SISEF - The Italian Society of Silviculture and Forest Ecology 2009
Open Access
This article is distributed under the terms of the Creative Commons Attribution-Non Commercial 4.0 International (https://creativecommons.org/licenses/by-nc/4.0/), which permits unrestricted use, distribution, and reproduction in any medium, provided you give appropriate credit to the original author(s) and the source, provide a link to the Creative Commons license, and indicate if changes were made.
Web Metrics
Breakdown by View Type
Article Usage
Total Article Views: 50214
(from publication date up to now)
Breakdown by View Type
HTML Page Views: 42056
Abstract Page Views: 3299
PDF Downloads: 3864
Citation/Reference Downloads: 123
XML Downloads: 872
Web Metrics
Days since publication: 6083
Overall contacts: 50214
Avg. contacts per week: 57.78
Article Citations
Article citations are based on data periodically collected from the Clarivate Web of Science web site
(last update: Mar 2025)
Total number of cites (since 2009): 4
Average cites per year: 0.24
Publication Metrics
by Dimensions ©
Articles citing this article
List of the papers citing this article based on CrossRef Cited-by.
Related Contents
iForest Similar Articles
Short Communications
The importance of forest type when incorporating forest edge deposition in the evaluation of critical load exceedance
vol. 2, pp. 43-45 (online: 21 January 2009)
Research Articles
Growth patterns of Scots pine (Pinus sylvestris L.) under the current regional pollution load in Lithuania
vol. 8, pp. 509-516 (online: 12 November 2014)
Research Articles
Deposition measurements and critical loads calculations: monitoring data, results and perspective
vol. 2, pp. 11-14 (online: 21 January 2009)
Research Articles
Fuel characterization and crown fuel load prediction in non-treated Calabrian pine (Pinus brutia Ten.) plantation areas
vol. 15, pp. 458-464 (online: 03 November 2022)
Short Communications
An approach to measuring biodiversity and its use in analysing the effect of nitrogen deposition on woodland butterfly populations in the Netherlands
vol. 2, pp. 46-48 (online: 21 January 2009)
Research Articles
Fuel consumption comparison of two forwarders in lowland forests of pedunculate oak
vol. 12, pp. 125-131 (online: 11 February 2019)
Research Articles
Estimation of fuel loads and carbon stocks of forest floor in endemic Dalmatian black pine forests
vol. 13, pp. 382-388 (online: 01 September 2020)
Commentaries & Perspectives
Clean air policy under the UNECE Convention on long-range transboundary air pollution: how are monitoring results “translated” to policy action
vol. 2, pp. 49-50 (online: 21 January 2009)
Research Articles
Acid atmospheric deposition in a forested mountain catchment
vol. 10, pp. 680-686 (online: 17 July 2017)
Research Articles
Nitrogen deposition and its impact on forest ecosystems in the Czech Republic - change in soil chemistry and ground vegetation
vol. 10, pp. 48-54 (online: 29 June 2016)
iForest Database Search
Search By Author
Search By Keyword
Google Scholar Search
Citing Articles
Search By Author
Search By Keywords
PubMed Search
Search By Author
Search By Keyword