The response of intra-annual stem circumference increase of young European beech provenances to 2012-2014 weather variability
iForest - Biogeosciences and Forestry, Volume 9, Issue 6, Pages 960-969 (2016)
doi: https://doi.org/10.3832/ifor1829-009
Published: Jun 23, 2016 - Copyright © 2016 SISEF
Research Articles
Collection/Special Issue: IUFRO RG7.01.00 - Nice (France 2015)
Global Challenges of Air Pollution and Climate Change to Forests
Guest Editors: Elena Paoletti, Pierre Sicard
Abstract
The increasing frequency and severity of extreme weather events, especially droughts, arising from on-going climate changes negatively affect productivity and stability of forest ecosystems. Understanding species responses and suitable ecotypes that are able of adapting to new environmental conditions is increasingly important. The objective of this study was to quantify the relationships between the inter-annual stem circumference increase (SCI) of five European beech (Fagus sylvatica L.) provenances and weather variability during 2012-2014 in a provenance trial located in central Slovakia. SCIs were extracted on daily and weekly scale from hourly data of circumference changes. To detect the main environmental factors influencing SCI seasonal dynamics, intra-seasonal moving correlation functions were calculated. All five provenances responded synchronously to weather conditions, with high correlations among them during the growing season on both daily and weekly scale. The photoperiod exhibited a synchronizing effect on the seasonal peak of SCI as a sign of tree adaptation to long-term seasonal variations in climate. Temperature was the most significant factor influencing SCI dynamics at the beginning of the season. During the summer months, a precipitation deficit, heat waves and the consequently decreased soil water potential significantly affected the SCI of young beech trees, despite the fact that the provenance plot was situated in an area of optimum beech growth. Not only the severity and duration were important but also the timing of drought within a season. Within all seasons, the lowest SCI values were recorded for the provenance from the lowest altitude and the most oceanic climate (northern Germany). A comparison of daily and weekly SCI with first derivatives of growth functions indicated that SCIs were closely related to theoretical incremental processes, especially on a weekly scale. In young beech trees, SCI seemed to represent an appropriate proxy for studying intra-seasonal incremental processes. A newly designed SASB (self adjusting sharp beginning) function fit these processes better than the Gompertz function.
Keywords
Stem Circumference Increase, Provenances, Fagus sylvatica, Weather Variables, Soil Water Potential
Introduction
European beech (Fagus sylvatica L.) covers an area of over 14 million ha in Europe ([49]), and 32.2% of the forested area in Slovakia ([1]). The increasing frequency and severity of extreme weather events, especially droughts, arising from on-going changes in climate have negatively affected the productivity and stability of forest ecosystems. Various studies pointed out the sensitivity of beech trees to prolonged drought ([14], [24]), mostly at its southern limits ([40]) or at Central European xeric sites ([5]). However, plastic responses of beech provenances to changes in environmental conditions ([42]) and a high species potential for different climates ([20]) have been reported.
Monitoring the dynamics of cambial activity and the development of woody cells ([13]), or measuring stem size variations throughout the season ([8]), are crucial metrics for understanding tree responses to seasonal environmental changes. When short measuring intervals are used ([8]), reversible shrinking and swelling of stems contribute to a significant part of the variability of stem size changes, reflecting the use of water stored in tree tissues ([4]). On the other hand, when using longer measuring intervals, shrinkage and swelling have a minor influence and dendrometers may provide accurate estimates of the timing of seasonal growth culmination and annual growth patterns ([3], [37]), especially in species like beech ([31], [44], [46]). The amount of newly formed wood can be understood as a suitable indicator for tree vigor or tree physiology ([6]). Growth traits represent a composite expression of underlying physiological mechanisms ([16]). Investigations into the seasonal dynamics of beech wood formation, especially concerning different provenances and their response to varying weather conditions in central Europe are insufficient.
Adaptive phenotypic plasticity enables plants to respond to environmental variability and is likely to buffer the impacts of climate change ([17]). Local adaptations to environmental conditions are of high ecological importance, as they determine distribution ranges and likely affect species responses to climate change ([27]). Introducing beech provenances from different environmental conditions may improve vigor, productivity or even stability and survival of beech ecosystems ([35], [11], [43]).
The primary objective of this study was to identify the main environmental factors, especially soil water availability and meteorological variables, that are linked to circumference increase dynamics in five central European beech provenances during three growing seasons. We hypothesized that: (1) weather fluctuations are reflected in seasonal circumference increases and that there are changes in their effects during the growing season; and (2) provenances differ in stem circumference increase.
Materials and methods
Experimental site and materials
This study is based on a provenance experiment with European beech established under the coordination of the Federal Forest Research Centre at the Institute of Forest Genetics in Grosshansdorf, Germany and was composed of 23 trials. The Slovak trial, located at Tále near the town of Zvolen in central Slovakia (Tab. 1, Fig. S1 in Supplementary material), composed of 32 provenances covering most of the distribution range of beech trees in Europe. The trial plot were planted in the year 1998 with 2-year-old seedlings. The test was established in a former forest nursery under a completely randomized block design with three blocks, each plot initially containing 50 plants planted with 2×1-m spacing ([15]).
Tab. 1 - Site information for the provenances used in the experiment and the experimental plot Tále. (T): long-term average yearly temperature; (Pr): long-term average yearly precipitation amount; (Ic): index of continentality.
| Code | Location | Country | Latitude | Longitude | Elevation (m a.s.l.) |
T (°C) |
Pr (mm) |
Ic (°C) |
|---|---|---|---|---|---|---|---|---|
| A | Postojna Javor | Slovenia | 14° 21′ N | 45° 44′ E | 1040 | 7.8 | 946 | 18.7 |
| B | Jaworze | Poland | 19° 10′ N | 49° 50′ E | 450 | 6.9 | 903 | 21.7 |
| C | Farchaus | Germany | 10° 40′ N | 53° 39′ E | 55 | 8.2 | 683 | 17.7 |
| D | Belzig | Germany | 12° 25′ N | 52° 03′ E | 140 | 8.7 | 557 | 18.4 |
| E | Eisenerz | Austria | 14° 51′ N | 47° 32′ E | 1100 | 4.2 | 1168 | 19.5 |
| - | Tále | Slovakia | 18° 59′ N | 48° 38′ E | 810 | 5.7 | 905 | 20.5 |
Tab. 2 - Descriptive statistics of the beech trees at the five locations. Diameter at breast height (DBH) in spring 2012 and height in autumn 2014.
| Location | Mean DBH ± STD (cm) |
DBH range (cm) |
Mean height ± STD (m) |
Height range (m) |
|---|---|---|---|---|
| Postojna Javor | 5.7 ± 1.1 | 4.4 - 7.4 | 7.0 ± 0.7 | 6.00 - 7.50 |
| Jaworze | 5.8 ± 0.6 | 5.3 - 7.0 | 6.9 ± 0.2 | 6.75 - 7.25 |
| Farchaus | 6.4 ± 1.3 | 5.3 - 8.0 | 6.9 ± 0.4 | 6.50 - 7.50 |
| Belzig | 6.6 ± 0.9 | 4.8 - 7.1 | 6.8 ± 0.5 | 6.50 - 7.50 |
| Eisenerz | 5.9 ± 1.0 | 4.7 - 7.3 | 6.9 ± 0.6 | 6.00 - 7.50 |
Out of each of five European provenances (Tab. 1, Fig. S1 in Supplementary material), six dominant trees were selected for measurement on micro-plots with 2 × 3 trees (3 trees on 2 micro-plots) experimental design during the 2012-2014 growing seasons. The measured trees were 14 years old in 2012 and their basic statistics are shown in Tab. 2. For the evaluation of competitive relationships, a Hegyi competition index ([33]) was calculated, where neighboring trees within 4 m of the subjects were considered as competitors (eqn. 1).
where DBHsi is the ith subject diameter, DBHcj is the jth competitor diameter and Lij is the subject-competitor distance in meters.
Meteorological data
Meteorological conditions were continuously monitored in an open plot in the center of the provenance plot. The conditions included air temperature (°C), relative humidity (%), global incoming solar radiation (W m-2 - Minikin TH®, EMS, Brno, CZ) and rainfall (mm - MetOne 370®, Oregon, USA). The measurements of soil water potential (Ψw) were carried out on each micro-plot at 15, 30 and 50 cm depth (continuously to -1.1 MPa, using gypsum blocks - Delmhorst Inc., USA - and MicroLog SP3 datalogger - EMS, Brno, CZ) and also on two micro-plots in the middle, near the meteo measurements. Overall, 36 gypsum blocks were used, 12 for each soil depth. We used the mean values of all of the blocks representing the whole site for the analyses to avoid mosaic heterogeneity between and inside the microplots.
Continentality was calculated as a simple index of continentality (Ic, [47] - eqn. 2):
where Tmax is the mean temperature (°C) of the warmest month and Tmin is the mean temperature (°C) of the coldest month.
The data of long term temperature averages for the Ic calculation and precipitation amounts (Tab. 1) for each geographic origin of the provenances were derived from WorldClim ([19]).
To calculate the average daily and weekly values used in seasonal growth dynamic analyses, the hourly meteo and Ψw values were shifted by 18 hours forward to correspond to the time of the circumference extraction (see below). Therefore, we calculated, e.g., the temperature until May 1st as the temperature in the 24 h preceding 6 AM. The weekly values until May 1st thus represent a 1-week (168 hours) average or sum (radiation, precipitation) before 6 AM on May 1st.
Stem circumference
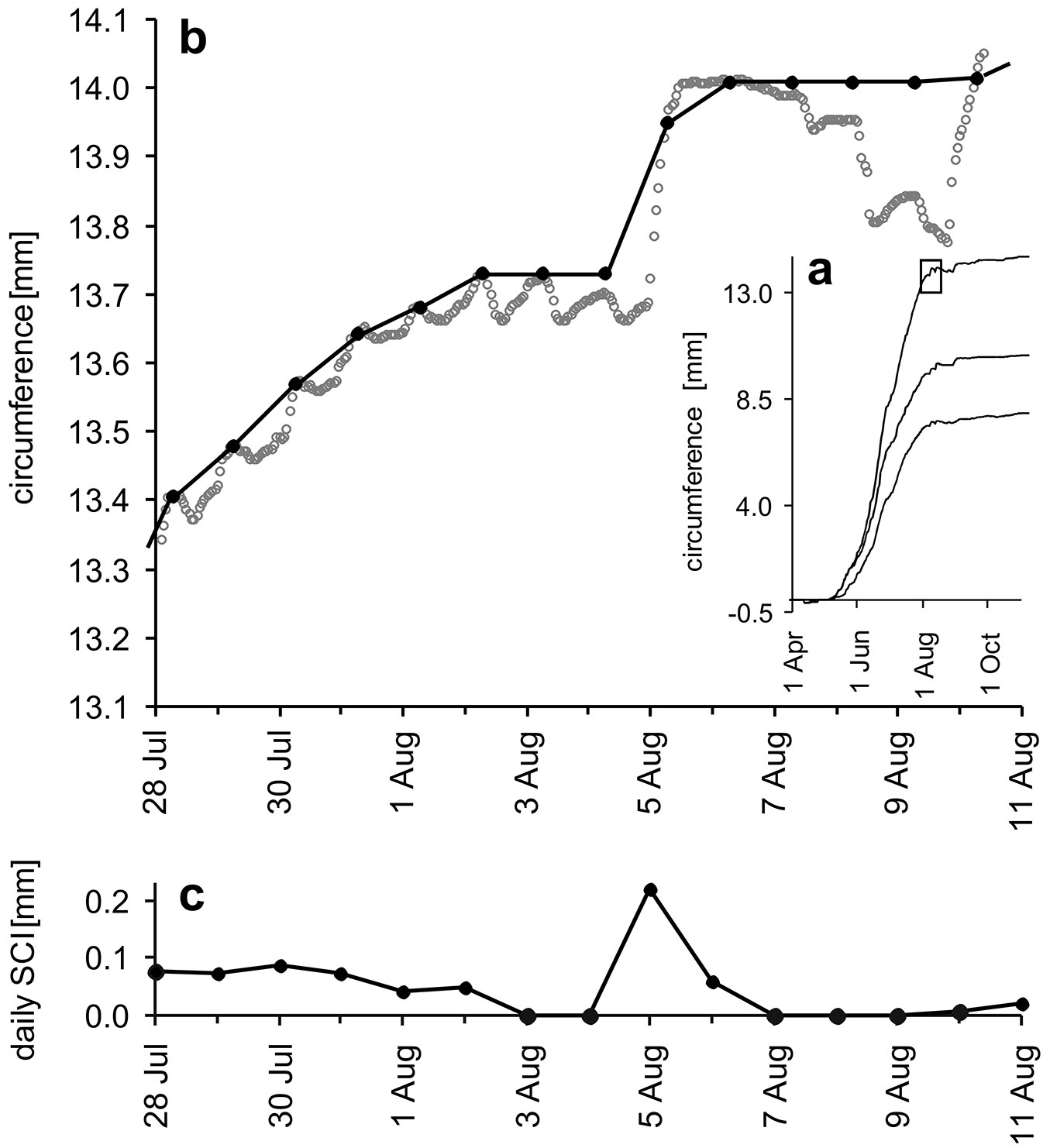
The changes in stem circumference were monitored using automatic band dendrometers (DRL, 26 application for small stems, EMS, Brno, CZ), which were non-invasively fixed to the trees at a height of 1.3 m. The hourly mean values were derived from measurements taken at 20-minute intervals. We calculated the daily stem circumference increase (SCI), extracted at 6 AM during the expansion phase of diurnal changes ([7], [23]), as the degree to which the morning 6 AM stem circumference exceeded the previous morning 6 AM maximum on the cumulative curve of seasonal stem circumference increases derived from the curve of 1 h circumference changes (Fig. 1). Weekly (or monthly) SCI for seasonal dynamics analyses were calculated as the sum of daily SCI during that period (Fig. 2).
Fig. 1 - The method of daily stem circumference increase (SCI) extraction. Seasonal curves of 1h stem circumference changes (a), rectangle indicates the part shown in detail (b), where: open grey circles represent 1 h circumferences, black line with solid black circles represents seasonal SCI at 6 CET in the morning which is situated on the plateau of expansion phase during the sunny days. During days when morning 6 CET circumferences were smaller than previous morning 6 CET maximum on the curve of stem circumferences, the previous maximum was used instead of them. Daily SCIs (c) represent the difference between two consecutive days on the curve of seasonal SCI in part b.
Fig. 2 - Seasonal dynamics of daily environmental variables and SCI. Average daily air temperature during 2012-2014 (a), average daily precipitation amounts (black bars) and average daily soil water potential (grey lines, vertical lines represent 95% confidence intervals) during 2012-2014 (b), average daily SCI of individual provenances (codes A-E are in Tab. 1) during 2012-2014 (c, d, e). R2 represents average inter-series R squared between provenances.
Cumulative daily and weekly SCI (growth curves) were fitted by the Gompertz (eqn. 3) and SASB (self adjusting sharp beginning - eqn. 4) functions using the software Mini32® (EMS Brno, CZ). The Gompertz function is defined in Rossi et al. ([36]) as (eqn. 3):
where y is the daily or weekly cumulative stem circumference increase, t is the time computed in Julian days, A is the upper asymptote of final cumulative stem circumference increase, β is the x-axis placement parameter, and k is the rate of change parameter.
The SASB growth function below was designed and long-term tested by EMS Brno in its software Mini32 (eqn. 4):
where (eqn. 5):
and y is the daily or weekly cumulative stem circumference increase, par1 is the starting value (offset, spring level of circumference), par2 is the annual growth, par3 is the time on maximal growth, par4 is the max growth rate, par5 is the virtual starting value (always lower than par1), and t is the time computed in Julian days.
The first derivatives of these functions were used for comparing extracted daily and weekly SCI values with theoretical incremental processes during the seasons.
Intra-seasonal moving correlation function
To analyze the main factors controlling circumference growth and changes and their effects during the season, we used an intra-seasonal moving correlation function ([22]).
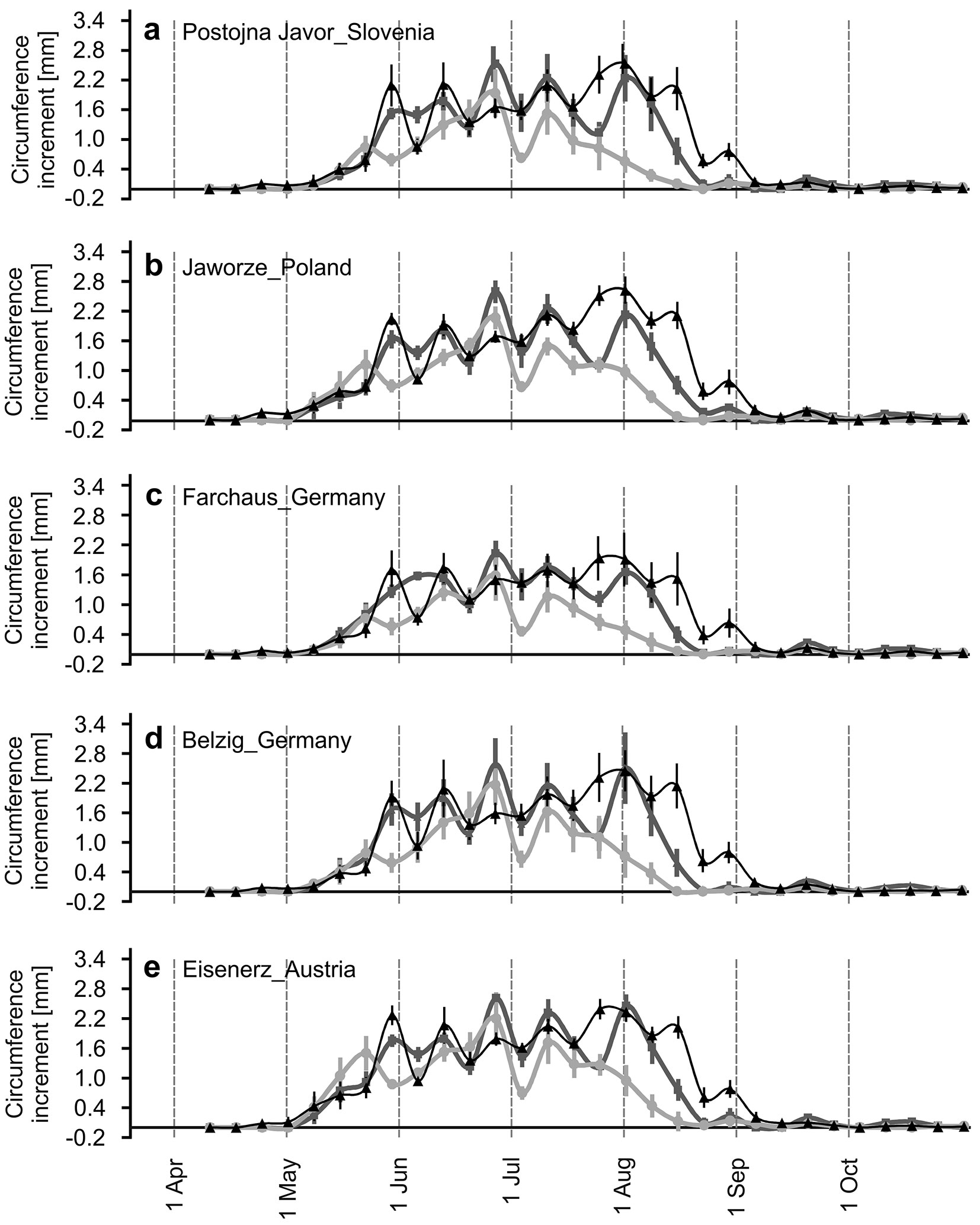
From the chronologies of circumference increments (e.g., Fig. 3a), time windows of 10 weeks (e.g., first from April 3 to June 5) were selected from each year and were joined chronologically (10 weeks from 2012 + 10 weeks from 2013 + 10 weeks from 2014) for both daily (n = 210) and weekly (n = 30) values.
Fig. 3 - Seasonal dynamics of weekly SCI. Average weekly SCI of individual provenances (a-e) in 2012 (bold dark-grey), 2013 (bold light-grey) and 2014 (fine black). Vertical lines represent 95% confidence intervals.
Additionally, the predictors were selected in the same way (daily or average weekly) minimal temperature (AT_min), daily (or average weekly) maximal temperature (AT_max), absolute weekly minimal temperature (AT_min a), absolute weekly maximal temperature (AT_max a), average daily (or weekly) temperature on an actual day (or week) (AT_avg), average weekly temperature one day (or week) preceding the actual day (or week - AT_p1), average daily (or weekly) temperature two days (or weeks) preceding the actual day (or week - AT_p2), average daily (or weekly) temperature three days (or weeks) preceding the actual day (or week - AT_p3), average daily (or weekly) air humidity (AH), average daily (or weekly) radiation (R), precipitation amount on the actual day (or week) (Pr), daily (or weekly) precipitation amount one day (or week) preceding the actual day (or week - Pr_p1), daily (or weekly) precipitation amount two days (or weeks) preceding the actual day (or week - Pr_p2), daily (or weekly) precipitation amount three days (or weeks) preceding the actual day (or week - Pr_p3), average daily (or weekly) soil water potential (Ψw) and average daily (or weekly) day length (DL).
The SCI chronologies were correlated with predictors. After this time, the windows were shifted 4 or 5 weeks (firstly to the period from May 1 to July 3 etc. - Fig. 3a-e) to cover the entire primary growth period.
Statistical analysis
Statistical analyses were performed using the statistical software Statistica® (Statsoft, Tulsa, OK, USA). Data were tested for normal distribution using Shapiro-Wilk’s W test. The significance of differences between the average values of annual SCI (Fig. S3 in Supplementary material) were tested using the Student’s t-test for independent samples. Based on the obtained results between all pairs of annual SCI, homogeneous groups at P < 0.05 were established.
Intra-seasonal moving correlation functions (Fig. 4, Fig. 5) were calculated using Spearman’s rank correlation coefficients, because a lot of SCI chronologies were not normally distributed. At the same time we supposed the relationships between measured SCI and weather factors to be monotonous (the higher is weather factor, the higher or lower is SCI), though not necessarily linear. For assessing such relationships, Spearman’s rank correlation coefficients are more suitable. However, the use of Pearson’s correlation coefficients provided similar results.
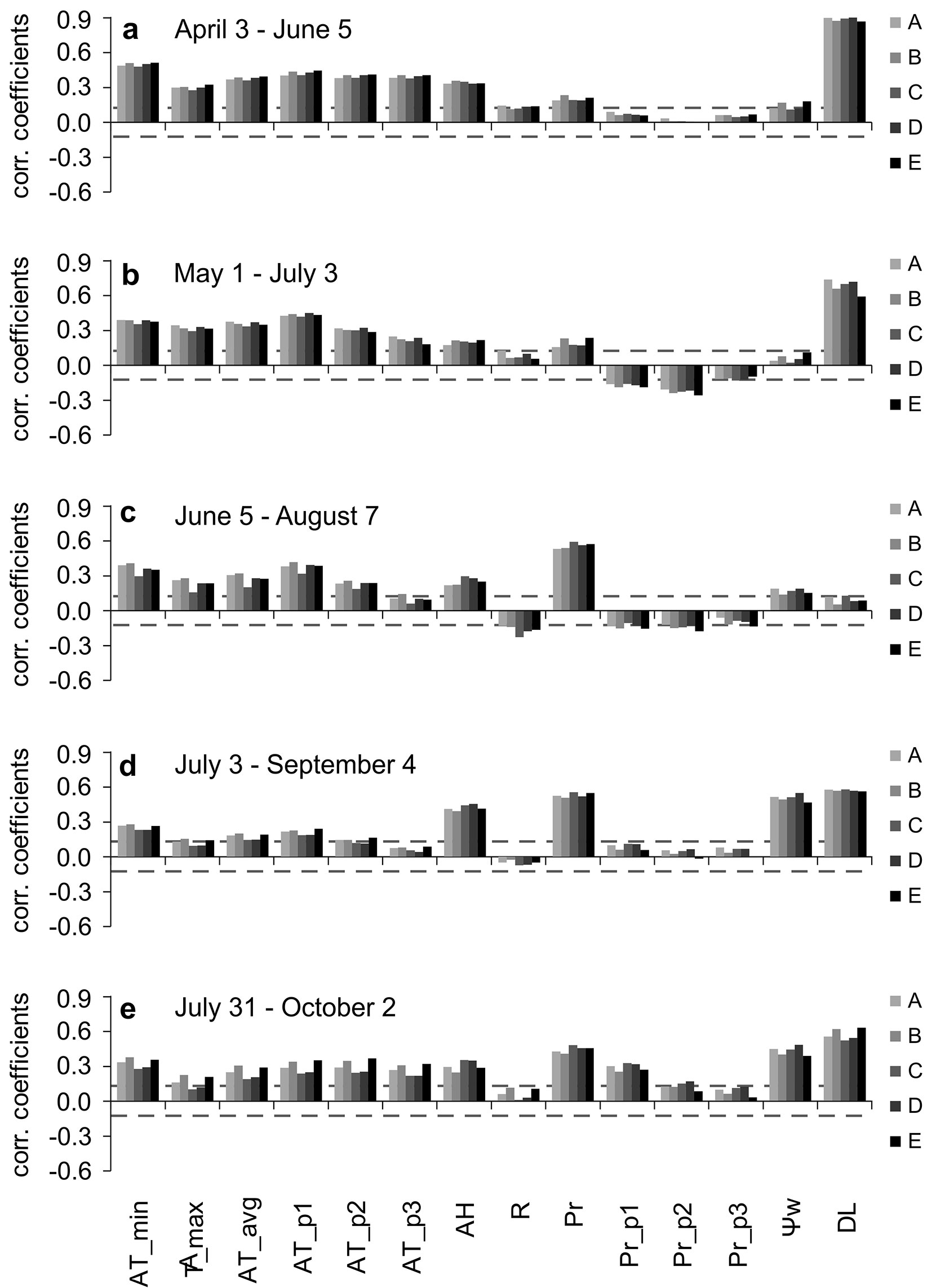
Fig. 4 - Results of intra-seasonal moving correlation functions. Correlations between daily SCI of individual provenances (codes A-E are in Tab. 1) and environmental variables in different periods (a-e) of the growing season. Correlation coefficients which meet dashed horizontal lines are statistically significant (P < 0.05). (AT_min): minimal daily temperature; (AT_max): maximal daily temperature, (AT_avg) average daily temperature on actual day, (AT_p1) average daily temperature one day preceding the actual day, (AT_p2) average daily temperature two days preceding the actual day; (AT_p3) average daily temperature three days preceding the actual day; (AH); average daily air humidity; (R): average daily radiation; (Pr): precipitation amount on an actual day; (Pr_p1): daily precipitation amount one day preceding the actual day; (Pr_p2): daily precipitation amount two days preceding the actual day; (Pr_p3): daily precipitation amount three days preceding the actual day; (Ψw): average daily soil water potential; (DL): average daily day length.
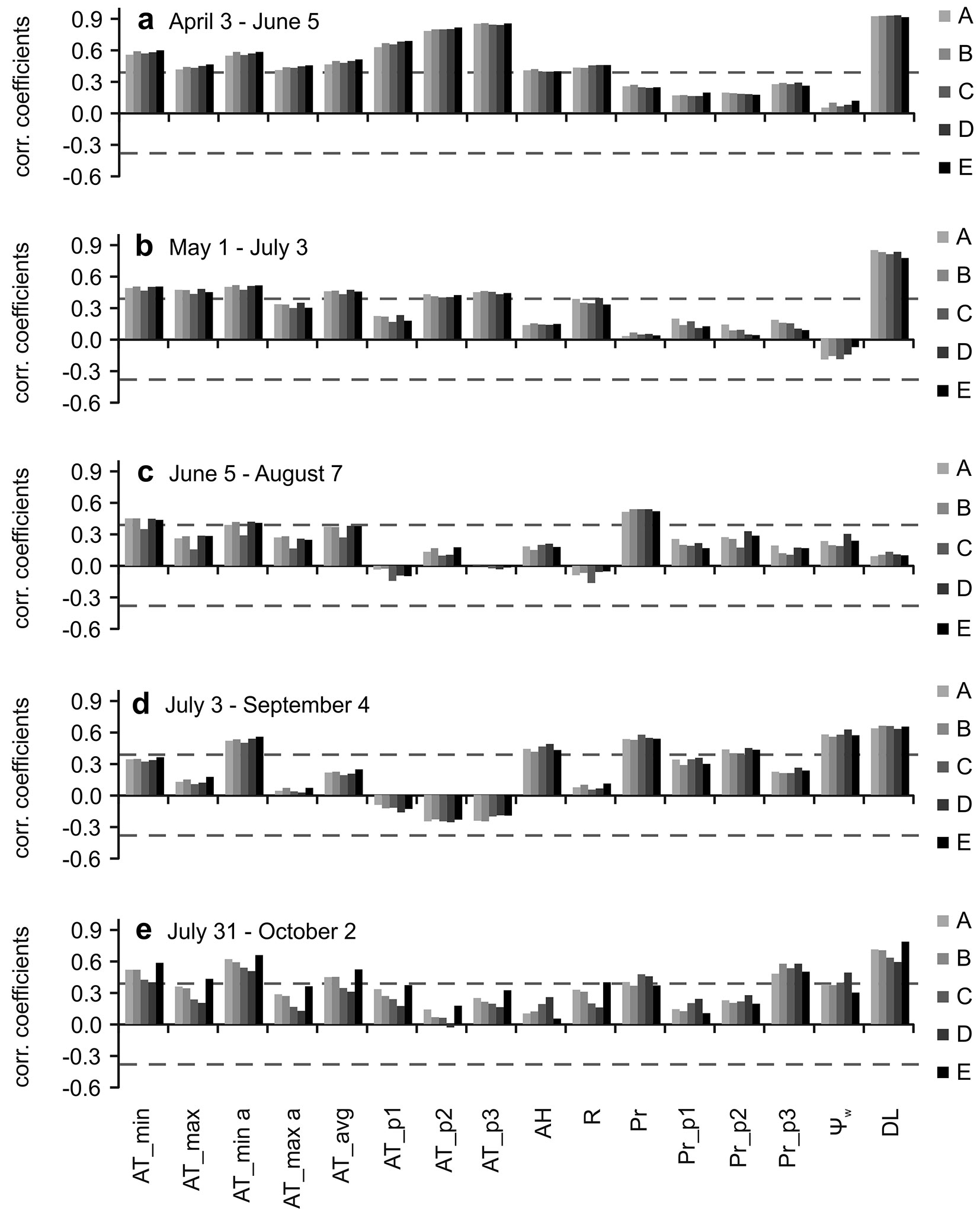
Fig. 5 - Results of intra-seasonal moving correlation functions. Correlations between weekly SCI of individual provenances (codes A-E are in Tab. 1) and environmental variables in different periods (a-e) of the growing season. Correlation coefficients which meet dashed horizontal lines are statistically significant (P < 0.05). (AT_min): average weekly minimal daily temperature; (AT_max): average weekly maximal daily temperature; (AT_min a): absolute weekly minimal temperature; (AT_max a): absolute weekly maximal temperature; (AT_avg): average weekly temperature on an actual week; (AT_p1): average weekly temperature one week preceding the actual week; (AT_p2): average weekly temperature two weeks preceding the actual week; (AT_p3): average weekly temperature three weeks preceding the actual week; (AH): average weekly air humidity; (R): average weekly radiation; (Pr): precipitation amount on an actual week; (Pr_p1): weekly precipitation amount one week preceding the actual week; (Pr_p2): weekly precipitation amount two weeks preceding the actual week; (Pr_p3): weekly precipitation amount three weeks preceding the actual week; (Ψw); average weekly soil water potential; (DL): average weekly day length.
Relations between 2012-2014 SCI and initial circumference, Hegyi competition index and continentality index mentioned above were calculated using Pearson’s correlation coefficients.
Results
Meteorology and soil water potential
While the second half of March and the beginning of April were relatively warm in 2012 and 2014, a long winter followed, lasting from December 2012 to 2013 (Fig. 2a, Fig. S2a in Supplementary material). This winter was terminated by rapid warming following the week of April 10th.
The subsequent growing seasons of 2012 and 2013 showed above average temperatures, including heat waves followed by some colder periods. There were no heat waves during 2014. Of particular contrast were the August temperatures of 3.3 °C and 3.5 °C higher than average in 2012 and 2013, respectively, compared to 2014. July and August 2014 had abundant precipitation (264 mm - Fig. S2b in Supplementary material) in contrast to the previous growing seasons, when 124 and 102 mm were recorded in 2012 and 2013, respectively.
These facts were reflected in decreased soil water potential (Ψw - Fig. 2b), which in 2014 moderately decreased at the end of June following the precipitation deficit, but then increased close to zero. During 2013, Ψw started to decrease by the end of the second decade of July followed by a marked decrease until a minimum on August 25th (-1.06 MPa), which then started to increase after August 26th and reached values close to zero after September 16th. During 2012, Ψw started to decrease by the end of the first week of August until the week of August 14th, then decreased to a minimum by September 12th (-0.97 MPa) and recovered after October 3rd.
Intraseasonal and interseasonal dynamics of stem circumference increase
The results indicate that all five provenances grew synchronously with high inter-correlations among them during a particular growing season (Fig. 2c-e, Fig. 3a-e) on both daily and weekly time scales.
Although in 2014 we registered the first SCI already between the weeks of April 17th - April 24th - May 1st, generally the “major growth period” began in the weeks between May 1st (Fig. 2c-e), May 8th (Fig. 3b, e, “Eisenerz” and “Jaworze” provenances) and May 15th (Fig. 3a, c, d) during all of the monitored years. The above-mentioned “major growth period” was characterized by marked fluctuations with ups and downs of weekly increment curves, which reached their seasonal peaks in the weeks of June 26th in 2012 and 2013 or in the week of July 24th or July 31st in 2014. The cessation of the “major growth period” could be assigned to the week of August 14th in 2013, August 21st in 2012 and September 4th in 2014. Despite the synchronous pattern of seasonal circumference increase, the provenances differed in the total amount of daily and weekly SCI. The highest SCI was recorded in the “Eisenerz” provenance, followed by the “Jaworze” and “Postojna Jawor”, the lowest occurred in the “Farchaus” provenance. These facts were also reflected in the yearly SCI (Fig. S3 in Supplementary material). The whole 2012-2014 circumference increase of the “Farchaus” provenance represented only 70% (-1.1 mm cm-1) of the “Eisenerz” provenance. In 2012 and 2014 it was 75% and 74% (-0.32 and 0.37 mm cm-1, Fig. S3 in Supplementary material), respectively. The greatest difference was recorded in 2013 (57%, -0.41 mm cm-1).
The selected groups of trees from individual provenances were homogeneous in their initial circumference values in 2012 (Fig. S4 in Supplementary material). No relationship was revealed between the initial circumferences and the 2012-2014 SCI (R2 = 0.000, P = 0.985) nor did one occur between the SCI and the Hegyi competition index (R2 = 0.000, P = 0.996).
Influence of weather factors on stem circumference increase
The intra-seasonal moving correlation function results indicate distinct seasonal variability in the influence from particular factors (Fig. 4a-e, Fig. 5a-e). The results confirm synchronous relationships in individual provenances between environmental factors and both the daily and weekly SCI.
At the beginning of the season, day length (DL) was the main factor promoting the increment on both time scales (Fig. 4a, Fig. 5a). The temperature in the actual, as well in the p1, p2 and p3 days or weeks positively impacted the SCI variability. Furthermore, especially minimum temperatures and air humidity and, on a weekly scale, radiation positively affected SCI as well.
In the second examined period, DL was again the main influencing factor (Fig. 4b, Fig. 5b). The effects of temperature and radiation were almost the same as in the previous period, except for lower correlations with previous weeks’ temperatures.
In the third period, the influence of DL disappeared, as it was near the summer solstice (Fig. 4c, Fig. 5c). The influence of temperature slightly decreased on a daily scale relative to the previous period, with the average temperature occurring 1 day prior the actual day as the most influencing, radiation and air humidity started to affect SCI slightly negatively and positively, respectively. The influence of radiation and temperature in previous weeks disappeared on the weekly scale. Precipitation on both scales started to positively affect the increments. On a daily scale, precipitation on the actual day became the main influencing factor.
During the fourth period, the correlations between SCI and DL were restored (Fig. 4d, Fig. 5d). Ψw became one of the main influencing factor on both daily and weekly scales. The influence of precipitation on previous weeks increased, as did the effect of air humidity. The effect of average temperatures during the actual week was slightly positive, while in previous weeks it was slightly negative. The highest correlations with temperatures were connected to the absolute minimum in the actual week. On a daily scale, the main influencing factor was precipitation, Ψw and DL followed by air humidity. In contrast to the weekly scale, the precipitation during preceding days did not positively influence SCI in accordance with previous periods.
In the fifth period, DL remained a positively influence on the daily and weekly SCI (Fig. 4e, Fig. 5e). The effect of Ψw, precipitation and air humidity decreased on both scales. The effects of temperature during the preceding days and weeks turned to a slightly positive influence and increased on a daily scale. Precipitation and Ψw became the main influencing weather variables on a daily scale. The absolute minimum temperatures in the actual week and precipitation in the p3 week became the main influencing weather variables on a weekly scale.
Overall, the largest differences between years were recorded in August, when individual provenances in 2013 formed only from 8 to 15% of the SCI in 2014, and from 41 to 52 % in 2012. The lack of precipitation and subsequent drought were manifested already in July in 2013, when the provenances formed from 44 to 60% of the SCI in 2014.
During all of the examined periods, the period between June 5th and August 7th was the most important for SCI formation when 75% to 78% of the total SCI for 2012-2014 was created.
We recorded a negative linear relationship (R2 = 0.91, P = 0.012) between the 2012-2014 SCI of the individual provenances and the absolute difference in continentality among the sites of origin and the Tále experimental plot.
Daily vs. weekly time scale of SCI
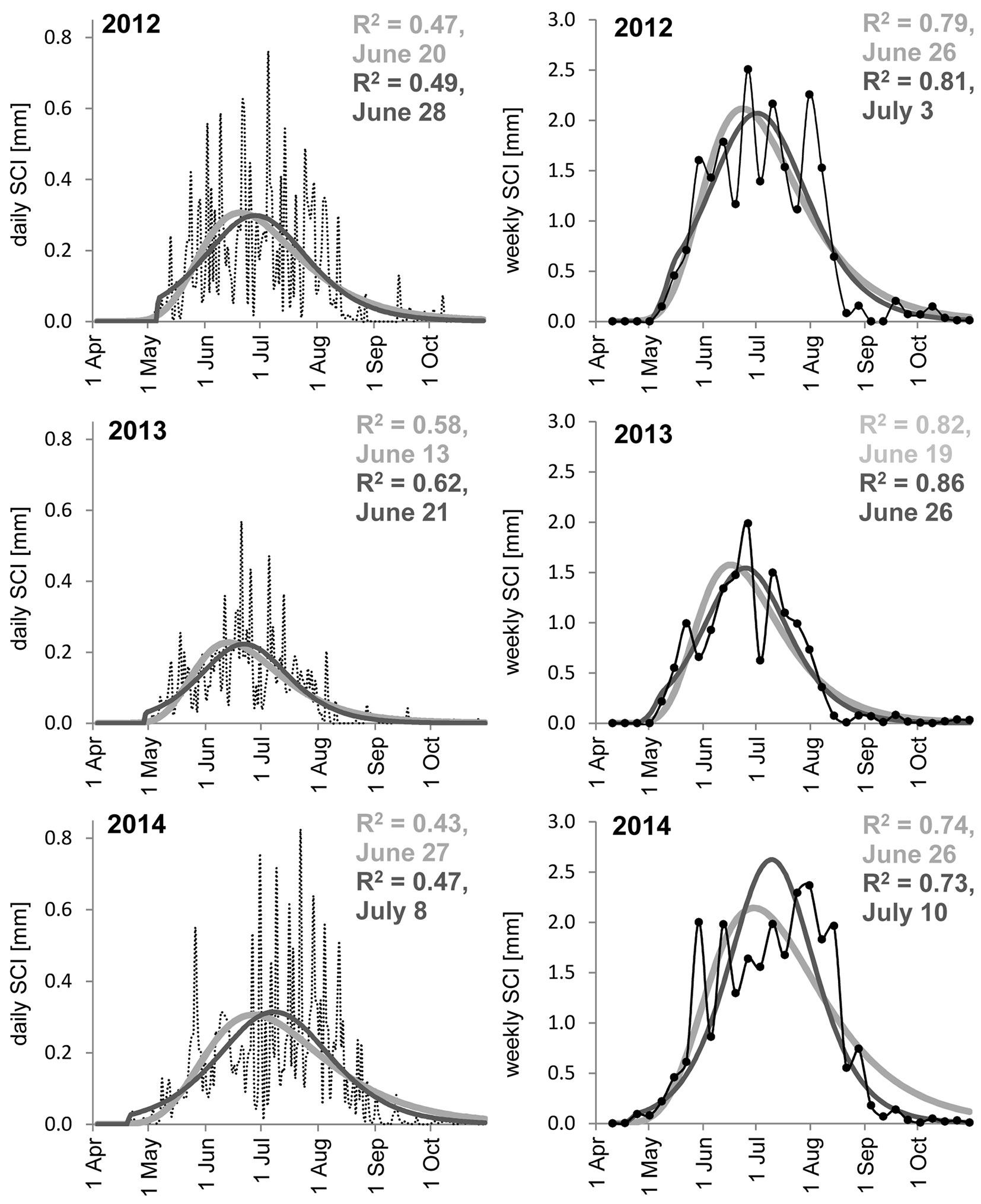
The weekly SCI were more linked to the theoretical incremental processes described by the first derivatives of the Gompertz (eqn. 3) and the newly defined SASB (eqn. 4) growth functions than the daily SCI (Fig. 6). At the same time, the SASB (eqn. 4) function fit more closely (higher R2) with either the daily or weekly values. The SASB function (eqn. 4) was originally designed for the abrupt start of growth. The SCI cessations were projected earlier, and their culmination was expected later, which fit more realistic recorded values.
Fig. 6 - Average daily (left, black dashed line) and weekly (right, black line with black circles) SCI of all studied trees (n=30) during 2012-2014 and their fit to the first derivatives of Gompertz (light grey) and SASB (dark grey) functions. R2 represent R squared between daily and weekly SCI and the first derivatives of growth functions; the date represent the date of culmination (day or week ending by day).
Discussion
Extraction of the SCI (increment) signal from the curves of circumference changes
The changes in tree stem size registered by dendrometers represent a complex mix of different environmental variables. Two basic components affect stem circumference (diameter, radius): the seasonal growth of stem tissues and variations in stem tissue water balance. Reversible changes are related to the fact that plants temporarily use water stored in tissues ([50]); therefore, the tissue-stored water serves as a water reservoir, buffering peaks of high water consumption that is reflected by diurnal or more prolonged pulsating changes in stem size ([4], [23]). This fact is considered to be a shortcoming of dendrometer measurements for the estimation of the seasonal dynamics of wood formation ([25]), especially for old, robust and slow-growing individuals or species with thick bark. At the Tále experimental site, we recorded clear diurnal courses of circumference changes and several-day shrinkage only during or close to drought events (Fig. 1a, b). This was probably the reason of the small initial size of tree stems and the relatively high seasonal growth during this stage. We used the daily SCI extracted at 6 AM during the expansion phase of diurnal changes ([7]) using a method where inter-morning shrinkage and recovery swelling were omitted. After finishing continuous courses of SCI related to drought events (Fig. 2c, d, e), only small SCI were recorded after rain events and soil water tension recovery in the autumn. These facts may be linked to the following: (i) some contractions and expansions of stems were still present in the data after SCI extraction and previous increases were overlapped by them; (ii) cell enlargement is far more sensitive to drought than cell division because cell turgor drives irreversible cell expansion and deposition of wall polymers ([34]), and the cell enlargement of some cells was delayed; or (iii) a combination of the both above-mentioned processes.
Furthermore, we compared the daily and weekly SCI for seasonal dynamics analyses. According to Bouriaud et al. ([3]) and Rossi et al. ([37]), shrinkage and swelling have a minor influence, with longer intervals between measurements and dendrometers may provide accurate estimates of the timing of seasonal growth culmination and the annual growth pattern, especially regarding species such as beech ([31], [44]). According to these statements, Ježík et al. ([21]) showed that high frequency data of daily circumference changes in beech trees close to their upper altitudinal limit were mostly linked to precipitation events, while low frequency variability was linked to temperature and its accumulation. Also Duchesne & Houle ([10]) reported that the high-frequency variation of stem diameter expansion was linked to rainy days. These facts were also supported by higher correlations with the first derivatives of the Gompertz (eqn. 3) and SASB (eqn. 4) growth functions (Fig. 6), using weekly SCI compared to daily SCI. However, the first derivatives of the growth functions were useful tools for approximating seasonal incremental processes; they were very smoothed and we may not assume that data derived from them were better than those extracted directly from dendrometers. They especially may not describe natural fluctuations (see Fig. 6, weekly courses on the right side) evidently linked to weather variations (e.g., temperature, not precipitation).
Hence, we believe that daily and particularly weekly SCI presented in young beech trees are appropriate proxies for studying intra-seasonal incremental processes.
Impact of environmental factors on intra- and inter-seasonal dynamics of stem circumference increase
Seasonal SCI of young beech trees is thus mainly linked to growth processes, consisting principally of two basic phases of cambial activity (cell division and enlargement) in the process of new tree-ring formation.
The long-term seasonal tree-growth biorhythm is partially synchronized with the photoperiod, not only because of its effects on basic physiological and growth processes ([18]) but also as a sign of local adaptations to a specific temperature-photoperiod regime ([39]). Note that, on a weekly scale, the correlations with day length are always higher than those with radiation measured directly on the plot (Fig. 5a-e). The synchronization of wood formation with photoperiod has been described for conifers at various sites in North America and Europe ([37]). At lower elevation sites, June seems to be an important month for beech growth due to the occurrence of the longest photoperiod ([6], [22]). At the Tále site, the highest SCI of all tree provenances were produced in June and July in 2012, in June in 2013 and in July in 2014. During August, SCI decreased owing to drought or sharp decreases in temperature, which probably interacted with decreasing photoperiods and caused cessation of growth. In 2014, SCI was high until August 14th (Fig. 2e, Fig. 3a-e, Fig. 6) following suitable conditions, with the next sharp decline linked to dropped temperatures (Fig. 2a). The correlations in Fig. 5d and Fig. 5e suggest that the minimal temperatures may be the main cause.
Various research papers ([2], [6]) report that beech increments began at the end of April and lasted until mid-May at lower elevation sites, reaching a peak from the end of May to mid-July, and ceased by mid-August or the end of August. These facts are in accordance with our findings, except culmination in 2014, which was shifted to the end of July. Leuzinger et al. ([29]), Werf et al. ([48]) Ježík et al. ([22]) and Van Der Maaten ([45]) reported that, as a result of a severe drought in 2003, the growth of beech trees was unusual in Europe. It already reached its peak in May-beginning of June, with further growth suppressed in the summer. Ježík et al. ([21]) noted that the seasonal diameter increase of beech close to its upper altitudinal limit in Slovakia was also correlated with temperature accumulation during previous weeks, as well as at a lower site at the beginning of the season ([22]). The results from the Tále experimental site also confirmed the importance of temperature and its accumulation, in addition to day-length at the beginning of the season (Fig. 5a, b). The temperature accumulation may also mask the influence of soil temperature, which is important for the beginning of xylogenesis ([38]).
Correlations indicate that during the summer, the effect of temperatures weakened (Fig. 4c, Fig. 5c), and during sufficient water availability, there was still linkage to temperature. This fact was well represented by a sharp drop in temperature and increments during the week of July 3rd, 2013 (Fig. 2a, d, Fig. 3a-e). During this week, the temperature decreased by -7.1 °C compared to a previous week, and the absolute minimal temperature reached only 3.5 °C. The importance of temperature during the summer was probably the result of higher elevation at the Tále site compared to most of the above-mentioned studies. It is situated in the beech growth optimum zone in Slovakia, only 5.9 km from the EES site ([22]), but 340 m higher in elevation, with milder summer temperatures and higher precipitation amounts. On the other hand, the correlations with temperatures at the beginning of the season (Fig. 5a, b) were smaller than those reported in Ježík et al. ([22]). This was probably a consequence of the fact that during all three studied seasons, the temperatures in April, May and June were considerably above the average, except in May 2014 (with only + 0.5 °C). However, winter 2014 was mild and warm in contrast to the winter of 2013, which ended at the beginning of April, followed by rapid warming (Fig. 2a).
Nevertheless, precipitation and soil water potential affected the SCI most significantly during the summer months (Fig. 4c, d, Fig. 5c, d). This fact resulted from a lack of precipitation, heat waves and decreased Ψw, mostly in 2013, when it occurred three weeks earlier than in 2012, at a time when high SCI could still be produced.
Drought can seriously affect the physiological and growth reactions of young beech trees ([35], [27]). According to Knutzen et al. ([26]), beech sapling morphology and root growth, in particular, responded to water shortage with higher phenotypic plasticity than physiology. The impact of precipitation, summer heat and water availability is well documented in dendrochronological studies at sites at lower elevations or close to the south range of their edge distribution ([9], [2], [28], [32], [12]). The course of seasonal daily and weekly SCI curves and their weather responses were synchronous between provenances, similar to the results by Stojnić et al. ([41]), which pointed to the environmental control of this trait ([11]). Similarly, the beech trees synchronously grew in different aspects in a study by Van Der Maaten ([45]).
Differences in the amount of SCI over the whole seasons among provenances were linked to differences between the continentality of sites of origin and provenance plot at the Tále site. A comparison of SCI with the summer heat moisture index ([43]), ecodistance ([30]), or timing of budburst or vegetation season length of individual provenances ([15]) did not identify so tight relationships.
Conclusions
The results indicated that stem circumference increase among all five provenances responded synchronously to weather conditions on a daily and weekly scale, with high inter-correlations among them during individual growing seasons. The photoperiod had a synchronizing effect on the seasonal culmination of SCI, which is a sign of tree adaptation to long-term seasonal variations in climate. Temperature was the most significant weather factor influencing the circumference increase dynamics at the beginning of the season and during the summer when soil water potential was high. During summer months, precipitation deficits, heat waves and consequently decreased soil water potential significantly affected the stem circumference increase of young beech trees, despite the fact that the provenance plot was situated in an area of optimal beech growth. While on a daily scale only precipitation on the actual day positively affected SCI, on a weekly scale precipitation in previous weeks was also important. Not only severity but also timing and duration of drought within the season were important. Within all seasons, the lowest increment was recorded at the provenance from the lowest altitude and the most oceanic climate (northern Germany).
A comparison of daily and weekly SCI values with the first derivatives of growth functions indicated that the weekly SCIs were closely related to theoretical incremental processes, suggesting that they can be recommended as appropriate proxy for studying intra-seasonal incremental processes in European beech.
List of abbreviations
The following abbreviations are used throughout the paper:
- AT_min: daily (or average weekly) minimal temperature
- AT_max: daily (or average weekly) maximal temperature
- AT_min a: absolute weekly minimal temperature
- AT_max a: absolute weekly maximal temperature
- AT_avg: average daily (or weekly) temperature on an actual day (or week)
- AT_p1: average weekly temperature one day (or week) preceding the actual day (or week)
- AT_p2: average daily (or weekly) temperature two days (or weeks) preceding the actual day (or week)
- AT_p3: average daily (or weekly) temperature three days (or weeks) preceding the actual day (or week)
- AH: average daily (or weekly) air humidity
- R: average daily (or weekly) radiation
- Pr: precipitation amount on an actual day (or week)
- Pr_p1: daily (or weekly) precipitation amount one day (or week) preceding the actual day (or week)
- Pr_p2: daily (or weekly) precipitation amount two days (or weeks) preceding the actual day (or week)
- Pr_p3: precipitation amount three days (or weeks) preceding the actual day (or week)
Ψw: average daily (or weekly) soil water potential- DL: average daily (or weekly) weekly day length
Acknowledgments
This work was supported by the Slovak Research and Development Agency (Contracts APVV 0135-12, APVV 0480-12) and the Grant Agency of the Ministry of Education and the Slovak Academy of Sciences (VEGA 0218-12, VEGA 0034-14 50 %).
References
Gscholar
CrossRef | Gscholar
CrossRef | Gscholar
CrossRef | Gscholar
Gscholar
Authors’ Info
Authors’ Affiliation
Miroslav Blaženec
Lubica Ditmarová
Institute of Forest Ecology, Slovak Academy of Sciences, Štúrova 2, 960 53 Zvolen (Slovak Republic)
EMS Brno, Turistická 5, 621 00 Brno (Czech Republic)
Faculty of Forestry, Technical University Zvolen, T. G. Masaryka 24, 960 53 Zvolen (Slovak Republic)
Corresponding author
Paper Info
Citation
Ježík M, Blaženec M, Kučera J, Strelcová K, Ditmarová L (2016). The response of intra-annual stem circumference increase of young European beech provenances to 2012-2014 weather variability. iForest 9: 960-969. - doi: 10.3832/ifor1829-009
Academic Editor
Elena Paoletti
Paper history
Received: Aug 29, 2015
Accepted: Mar 11, 2016
First online: Jun 23, 2016
Publication Date: Dec 14, 2016
Publication Time: 3.47 months
Copyright Information
© SISEF - The Italian Society of Silviculture and Forest Ecology 2016
Open Access
This article is distributed under the terms of the Creative Commons Attribution-Non Commercial 4.0 International (https://creativecommons.org/licenses/by-nc/4.0/), which permits unrestricted use, distribution, and reproduction in any medium, provided you give appropriate credit to the original author(s) and the source, provide a link to the Creative Commons license, and indicate if changes were made.
Web Metrics
Breakdown by View Type
Article Usage
Total Article Views: 50418
(from publication date up to now)
Breakdown by View Type
HTML Page Views: 42004
Abstract Page Views: 3075
PDF Downloads: 3963
Citation/Reference Downloads: 57
XML Downloads: 1319
Web Metrics
Days since publication: 3375
Overall contacts: 50418
Avg. contacts per week: 104.57
Article Citations
Article citations are based on data periodically collected from the Clarivate Web of Science web site
(last update: Mar 2025)
Total number of cites (since 2016): 16
Average cites per year: 1.60
Publication Metrics
by Dimensions ©
Articles citing this article
List of the papers citing this article based on CrossRef Cited-by.
Related Contents
iForest Similar Articles
Research Articles
Adaptive variation in physiological traits of beech provenances in Central Europe
vol. 11, pp. 24-31 (online: 09 January 2018)
Research Articles
Identification and molecular characterization of LTR and LINE retrotransposable elements in Fagus sylvatica L.
vol. 2, pp. 119-126 (online: 10 June 2009)
Short Communications
Growth of Stone pine (Pinus pinea L.) European provenances in central Chile
vol. 10, pp. 64-69 (online: 29 August 2016)
Research Articles
Suitability of Fagus orientalis Lipsky at marginal Fagus sylvatica L. forest sites in Southern Germany
vol. 15, pp. 417-423 (online: 19 October 2022)
Research Articles
Patterns of genetic diversity in European beech (Fagus sylvatica L.) at the eastern margins of its distribution range
vol. 10, pp. 916-922 (online: 10 December 2017)
Short Communications
Differential adaptations in nursery seedlings from diverse Chilean provenances of Peumus boldus Mol.
vol. 9, pp. 409-413 (online: 29 January 2016)
Research Articles
Influence of tree water potential in inducing flowering in Rhododendron arboreum in the central Himalayan region
vol. 9, pp. 842-846 (online: 06 May 2016)
Research Articles
Long-term effects of stem girdling on needle structure in Scots pine
vol. 11, pp. 476-481 (online: 02 July 2018)
Research Articles
Soil water deficit as a tool to measure water stress and inform silvicultural management in the Cape Forest Regions, South Africa
vol. 13, pp. 473-481 (online: 01 November 2020)
Research Articles
Juvenile growth response of European beech (Fagus sylvatica L.) to sudden change of climatic environment in SE European trials
vol. 2, pp. 213-220 (online: 22 December 2009)
iForest Database Search
Search By Author
Search By Keyword
Google Scholar Search
Citing Articles
Search By Author
Search By Keywords
PubMed Search
Search By Author
Search By Keyword